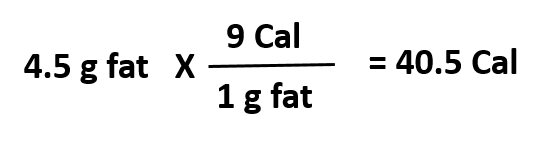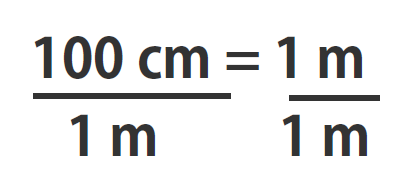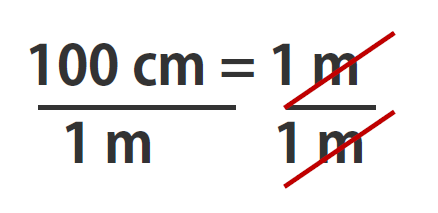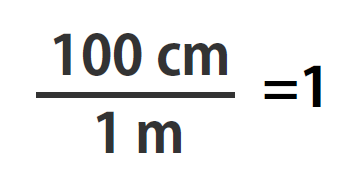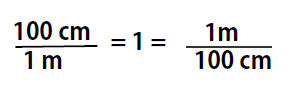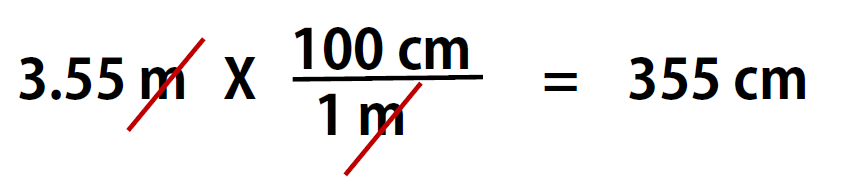Home » Student Resources » Online Chemistry Textbooks » CH103: Allied Health Chemistry » CH103 – CHAPTER 1: Math for Allied Health Chemistry
MenuCH103: Allied Health Chemistry
Chapter 1: Math for Allied Health Chemistry
This text is published under creative commons licensing. For referencing this work, please click here.
Section 1.1: Scientific Notation
Video Tutorial
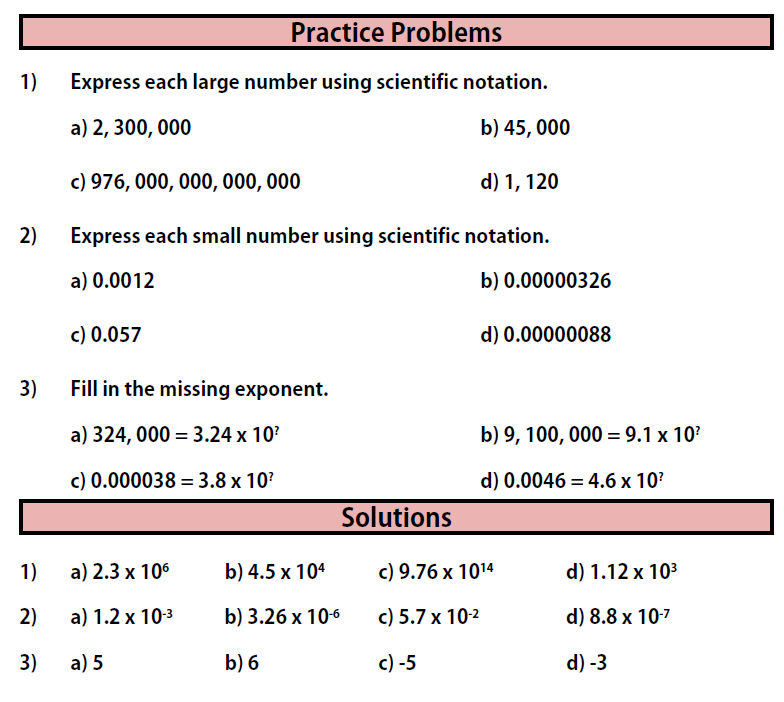
Practice Problems
Section 1.2: Units of Measurement
International System of Units and the Metric System
Derived SI Units
Drop Units
Section 1.3: Making Measurements in the Lab
Precision vs. Accuracy
Significant Figures
Exact Numbers
Rules of Rounding
Video Tutorial
Calculations with Significant Figures
Conversions and the Importance of Units
Conversion Factors
1.4 Chapter Summary
1.5 Chapter Homework
1.6 References
In this chapter, you will find a review of the major mathematical considerations needed to be successful in this course.
1.1: Scientific Notation
The study of chemistry can involve numbers that are very large. It can also involve numbers that are very small. Writing out such numbers and using them in their long form is problematic, because we would spend far too much time writing zeroes, and we would probably make a lot of mistakes! There is a solution to this problem. It is called scientific notation.
Scientific notation allows us to express very large and very small numbers using powers of 10.
Recall that:
100 = 1 101 = 10 102 = 100
103 = 1000 104 = 10000 105 = 100000
As you can see, the power to which 10 is raised is equal to the number of zeroes that follow the 1. This will be helpful for determining which exponent to use when we express numbers using scientific notation.
Let us take a very large number:
579, 000, 000, 000
and express it using scientific notation.
First, we find the coefficient, which is a number between 1 and 10 that will be multiplied by 10 raised to some power.
Our coefficient is: 5.79
This number will be multiplied by 10 that is raised to some power. Now let us figure out what power that is.
We can do this by counting the number of positions that stand between the end of the original number and the new position of the decimal point in our coefficient.
5 . 7 9 0 0 0 0 0 0 0 0 0
↑ ↑
How many positions are there?
We can see that there are 11 positions between our decimal and the end of the original number. This means that our coefficient, 5.79, will be multiplied by 10 raised to the 11th power.
Our number expressed in scientific notation is:
5.79 x 1011
But what about very small numbers?
You may recall that:
10-1 = 0.1 10-2 = 0.01 10-3 = 0.001
10-4 = 0.0001 10-5 = 0.00001
The number of spaces to the right of the decimal point for our 1 is equal to the number in the exponent that is behind the negative sign. This is useful to keep in mind when we express very small numbers in scientific notation.
Here is a very small number:
0.0000642
Let us express this number using scientific notation.
Our coefficient will be 6.42
This number will be multiplied by 10 raised to some power, which will be negative. Let us figure out the correct power. We can figure this out by counting how many positions stand between the decimal point in our coefficient and the decimal point in our original number.
0 . 0 0 0 0 6 4 2
↑ ↑
How many positions?
There are 5 positions between our new decimal point and the decimal point in the original number, so our coefficient will be multiplied by 10 raised to the negative 5th power.
Our number written in scientific notation is:
6.42 x 10-5
You can use these methods to express any large or small number using scientific notation.
VIDEO TUTORIAL FOR SIGNIFICANT FIGURES:
Practice Problems for Section 3
Quiz Yourself: More Practice with Scientific Notation
1.2: Units of Measurement
International System of Units and the Metric System
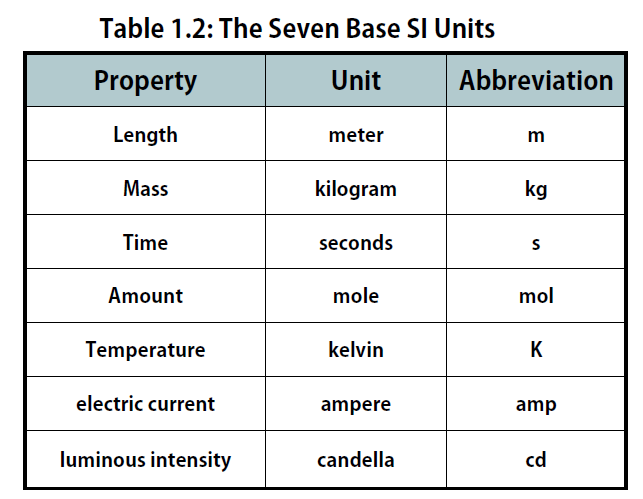
The International System of Units, abbreviated SI from the French Système International D’unités, is the main system of measurement units used in science. Since the 1960s, the International System of Units has been internationally agreed upon as the standard metric system. The SI base units are based on physical standards. The definitions of the SI base units have been and continue to be modified and new base units added as advancements in science are made. Each SI base unit except the kilogram is described by stable properties of the universe.
There are seven base units, which are listed in Table 1.2. Chemistry primarily uses five of the base units: the mole for amount, the kilogram for mass, the meter for length, the second for time, and the kelvin for temperature. The degree Celsius (oC) is also commonly used for temperature. The numerical relationship between kelvins and degrees Celsius is as follows
K = oC + 273
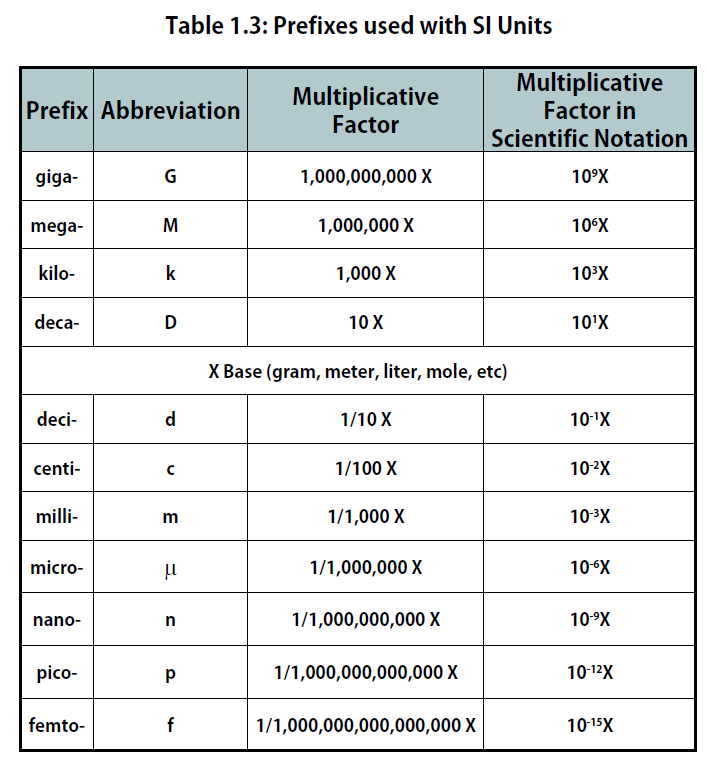
The size of each base unit is defined by international convention. For example, the kilogram is defined as the quantity of mass of a special metal cylinder kept in a vault in France (Figure 1.1). The other base units have similar definitions. The sizes of the base units are not always convenient for all measurements. For example, a meter is a rather large unit for describing the width of something as narrow as human hair. Instead of reporting the diameter of hair as 0.00012 m or even 1.2 × 10-4 m, SI also provides a series of prefixes that can be attached to the units, creating units that are larger or smaller by powers of 10, known as the metric system.
Figure 1.1 The Kilogram. The standard for the kilogram is a platinum-iridium cylinder kept in a speacial vault in France. Source: Wikimedea (https://commons.wikimedia.org/wiki/File:National_prototype_kilogram_K20_replica.jpg)
Common prefixes and their multiplicative factors are listed in Table 1.3 “Prefixes Used with SI Units”. (Perhaps you have already noticed that the base unit kilogram is a combination of a prefix, kilo- meaning 1,000 ×, and a unit of mass, the gram.) Some prefixes create a multiple of the original unit: 1 kilogram equals 1,000 grams (or 1 kg = 1,000 g), and 1 megameter equals 1,000,000 meters (or 1 Mm = 1,000,000 m). Other prefixes create a fraction of the original unit. Thus, 1 centimeter equals 1/100 of a meter, 1 millimeter equals 1/1,000 of a meter, 1 microgram equals 1/1,000,000 of a gram, and so forth.
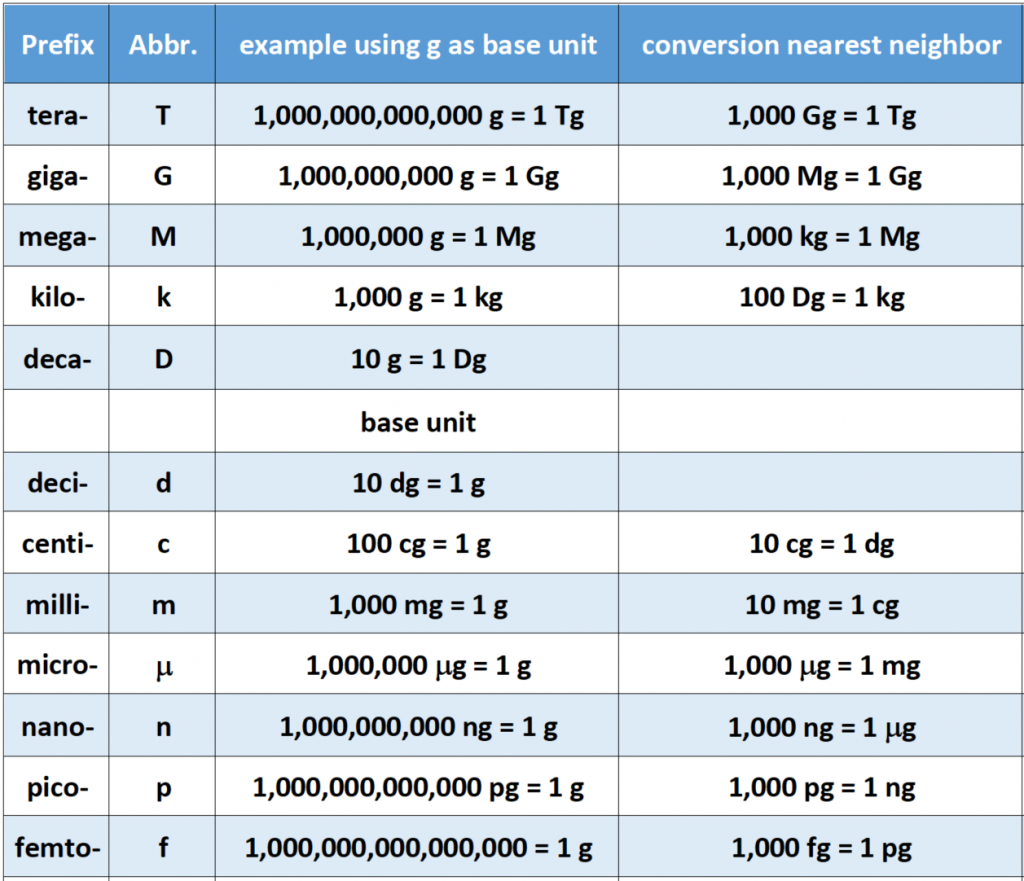
sometimes this chart can be a little difficult to effectively use when preparing your conversion factors. Thus, Table 1.3a below gives an example of the correct conversions using the gram as the base unit. In addition, the larger unit, tera- is also included.
Table 1.3a Example of Metric Conversions Using the Gram as the Base Unit
The basic unit of mass in the International System of Units is the kilogram. A kilogram is equal to 1000 grams. A gram is a relatively small amount of mass and so larger masses are often expressed in kilograms. When very tiny amounts of matter are measured, we often use milligrams which are equal to 0.001 gram. There are numerous larger, smaller, and intermediate mass units that may also be appropriate. At the end of the 18th century, a kilogram was the mass of a liter of water. In 1889, a new international prototype of the kilogram was made of a platinum-iridium alloy. The kilogram is equal to the mass of this international prototype, which is held in Paris, France.
Mass and weight are not the same thing. Although we often use the terms mass and weight interchangeably, each one has a specific definition and usage. The mass of an object is a measure of the amount of matter in it. The mass (amount of matter) of an object remains the same regardless of where the object is placed. For example, moving a brick to the moon does not cause any matter in it to disappear or be removed.
The weight of an object is determined by the force that gravitation exerts upon the object. The weight is equal to the mass of the object times the local acceleration of gravity. Thus, on the Earth, weight is determined by the force of attraction between the object and the Earth. Since the force of gravity is not the same at every point on the Earth’s surface, the weight of an object is not constant. The gravitational pull on the object varies depending on where the object is with respect to the Earth or other gravity-producing object. For example, a man who weighs 180 pounds on Earth would weigh only 45 pounds if he were in a stationary position, 4,000 miles above the Earth’s surface. This same man would weigh only 30 pounds on the moon because the moon’s gravity is only one-sixth that of Earth. The mass of this man, however, would be the same in each situation. For scientific experiments, it is important to measure the mass of a substance rather than the weight to retain consistency in the results regardless of where you are performing the experiment.
Length
The SI unit of length is the meter. In 1889, the definition of the meter was a bar of platinum-iridium alloy stored under conditions specified by the International Bureau of Standards. In 1960, this definition of the standard meter was replaced by a definition based on a wavelength of krypton-86 radiation. In 1983, that definition was replaced by the following: the meter is the length of the path traveled by light in a vacuum during a time interval of a second.
Temperature
When used in a scientific context, the words heat and temperature do NOT mean the same thing. Temperature represents the average kinetic energy of the particles that make up a material. Increasing the temperature of a material increases its thermal energy. Thermal energy is the sum of the kinetic and potential energy in the particles that make up a material. Objects do not “contain” heat; rather they contain thermal energy. Heat is the movement of thermal energy from a warmer object to a cooler object. When thermal energy moves from one object to another, the temperature of both objects change.
A thermometer is a device that measures temperature. The name is made up of “thermo” which means heat and “meter” which means to measure. The temperature of a substance is directly proportional to the average kinetic energy it contains. In order for the average kinetic energy and temperature of a substance to be directly proportional, it is necessary that when the temperature is zero, the average kinetic energy must also be zero. It was necessary for use in calculations in science for a third temperature scale in which zero degrees corresponds with zero kinetic energy, that is, the point where molecules cease to move. This temperature scale was designed by Lord Kelvin. Lord Kelvin stated that there is no upper limit of how hot things can get, but there is a limit as to how cold things can get. In 1848, William Lord Kelvin developed the idea of absolute zero, which is the temperature at which molecules stop moving and therefore, have zero kinetic energy. This is known as the Kelvin temperature scale.
The Celsius scale is based on the freezing point and boiling point of water. Thus, 0oC is the freezing point of water, whereas 100oC is the boiling point of water. Most of us are familiar with temperatures that are below the freezing point of water. It should be apparent that even though the air temperature may be -5oC, the molecules of air are still moving (i.e. 0oC is not absolute zero). Substances like oxygen gas and nitrogen gas have already melted and boiled to vapor at temperatures below -150oC.
The Fahrenheit scale is also defined by the freezing point and boiling points of water. However, the scale is different from that of the Kelvin and Celsius scales. In the Fahrenheit scale, the freezing point of water is 32oF and the boiling point of water is 212oF. To convert between the Fahrenheit scale and the Celsius scale , the following conversions can be used:
[oC] = ([oF] -32) × 5/9 or [oF] = [oC] × 9/5 + 32
The Kelvin temperature scale has its zero at absolute zero (determined to be -273.15oC), and uses the same degree scale as the Celsius scale. Therefore, the mathematical relationship between the Celsius scale and the Kelvin scale is
K = oC + 273.15
In the case of the Kelvin scale, the degree sign is not used. Temperatures are expressed simply as 450 K, and are always positive.
Time
The SI unit for time is the second. The second was originally defined as a tiny fraction of the time required for the Earth to orbit the Sun. It has since been redefined several times. The definition of a second (established in 1967 and reaffirmed in 1997) is: the duration of 9,192,631,770 periods of the radiation corresponding to the transition between the two hyperfine levels of the ground state of the cesium-133 atom.
Amount
Chemists use the term mole to represent a large number of atoms or molecules. Just as a dozen implies 12 things, a mole (mol) represents 6.022 × 1023 things. The number 6.022 × 1023, called Avogadro’s number after the 19th-century chemist Amedeo Avogadro, is the number we use in chemistry to represent macroscopic amounts of atoms and molecules. Thus, if we have 6.022 × 1023 Oxygen atoms, we say we have 1 mol of Oxygen atoms. If we have 2 mol of Na atoms, we have 2 × (6.022 × 1023) Na atoms, or 1.2044 × 1024 Na atoms. Similarly, if we have 0.5 mol of benzene (C6H6) molecules, we have 0.5 × (6.022 × 1023) C6H6 molecules, or 3.011 × 1023 C6H6 molecules.
Derived SI Units
Derived units are combinations of SI base units. Units can be multiplied and divided, just as numbers can be multiplied and divided. For example, the area of a square having a side of 2 cm is 2 cm × 2 cm, or 4 cm2 (read as “four centimeters squared” or “four square centimeters”). Notice that we have squared a length unit, the centimeter, to get a derived unit for area, the square centimeter.
Volume
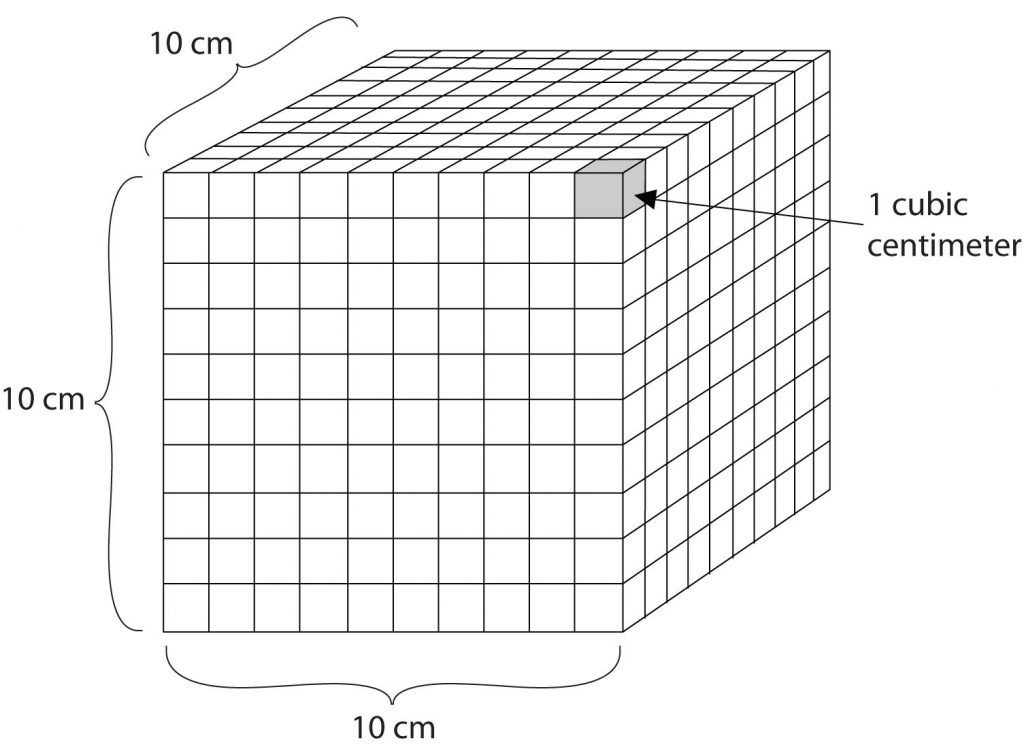
Volume is an important quantity that uses a derived unit. Volume is the amount of space that a given substance occupies and is defined geometrically as length × width × height. Each distance can be expressed using the meter unit, so volume has the derived unit m × m × m, or m3 (read as “meters cubed” or “cubic meters”). A cubic meter is a rather large volume, so scientists typically express volumes in terms of 1/1,000 of a cubic meter. This unit has its own name—the liter (L). A liter is a little larger than 1 US quart in volume. (Table 1.4) gives approximate equivalents for some of the units used in chemistry.) As shown in Figure 1.3 “The Liter”, a liter is also 1,000 cm3. By definition, there are 1,000 mL in 1 L, so 1 milliliter and 1 cubic centimeter represent the same volume.
1 mL = 1 cm3
Figure 1.3: The Liter. A liter is defined as a cube that is 10 cm (1/10th of a meter) on a side. A milliliter, 1/1000th of a liter, is equal to 1 cubic centimeter (1 cm3).
Energy
Energy, another important quantity in chemistry, is the ability to perform work. Moving a box of books from one side of a room to the other side, for example, requires energy. It has a derived unit of kg·m2/s2. (The dot between the kg and m2 units implies the units are multiplied together and then the whole term is divided by s2.) Because this combination is cumbersome, this collection of units is redefined as a joule (J), which is the SI unit of energy. An older unit of energy, the calorie (cal), is also widely used. There are:
4.184 J = 1 cal
Note that this differs from our common use of the big ‘Calorie’or ‘Cal’ listed on food packages in the United States. The big ‘Cal’ is actually a kilocalorie or kcal (Fig 1.4) Note that all chemical processes or reactions occur with a simultaneous change in energy and that energy can be stored in chemical bonds. We can think of this in terms of our food molecules. The chemical bonds in proteins, carbohydrates, and fats store a large amount of energy that our bodies can harvest and convert into usable forms of energy to power brain function, muscle contraction, and build new bone, muscle, and other tissues. This energy can be calculated with proteins yielding 4 Cal/g (4 kcal/g), carbohydrates yielding 4 Cal/g (4 kcal/g), and fats yielding 9 Cal/g (9 kcal/g).
Figure 1.4: The Difference between kilocalories in Scientific and Common Use. Calories represented on food packaging actually refer to kilocalories in scientific terms.
Density
Density is defined as the mass of an object divided by its volume; it describes the amount of matter contained in a given amount of space.
density=mass/volume
Thus, the units of density are the units of mass divided by the units of volume: g/cm3 or g/mL (for solids and liquids, respectively), g/L (for gases), kg/m3, and so forth. For example, the density of water is about 1.00 g/mL, while the density of mercury is 13.6 g/mL. Mercury is over 13 times as dense as water, meaning that it contains over 13 times the amount of matter in the same amount of space. The density of air at room temperature is about 1.3 g/L.
Drop Units
Within a hospital setting, medications are often delivered by intravenous fluids (IV). This means that dosages are often calculated by using the number of drops delivered per mL of solution over time. The abbreviation gtt is used to denote drop and comes from the Latin word for drop which is gutta. The drop factor (gtt/mL) can be used to determine drip rates when a prescribed volume of medicine is required over a given time period. Note that drop factors will vary depending on the diameter of the IV tubing that is being used.
1.3: Making Measurements in the Lab
Precision vs. Accuracy
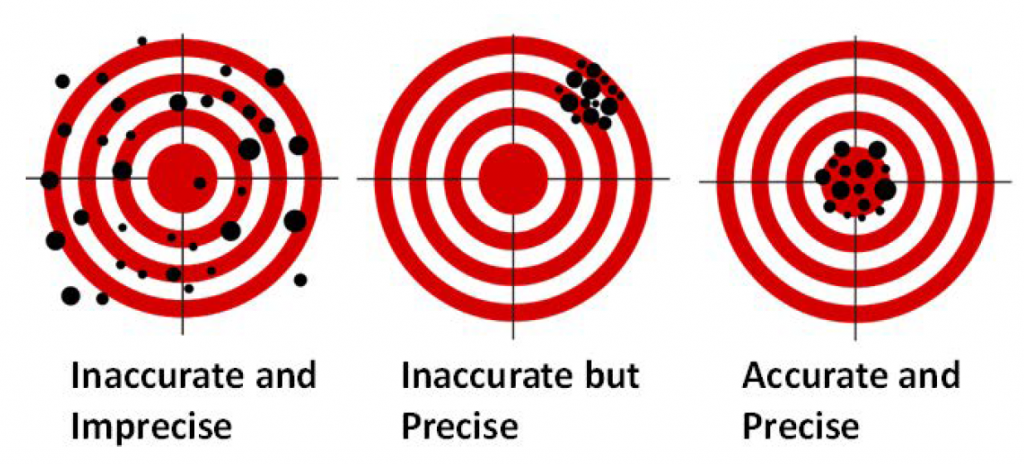
It is important to note the different terminology we use when talking in science. One such set of terminology is precision and accuracy. Although precision and accuracy are often used interchangeably in the non-scientific community, the difference between the terms is extremely important to realize. Precision tells you how close two measurements are to one another, while accuracy tells you how close a measurement is to the known value. A measurement can be precise while not being accurate, or accurate but not precise; the two terms are NOT related. A good analogy can be found in a game of darts (Fig. 1.5). A player who always hits the same spot just to the left of the dart board would be precise but not very accurate. However, a dart player who is all over the board but hits the center of the board on average would be accurate but not precise. A good darts player, just like a good scientist, wants to be both precise and accurate.
Figure 1.5: Difference Between Accuracy and Precision. A game of darts can be used to show the difference between accuracy and precision.
Adapted from: https://upload.wikimedia.org/wikipedia/commons/thumb/5/5d/Reliability_and_validity.svg/717px-Reliability_and_validity.svg.png
Typically within the laboratory, accuracy is a measure of how well your equipment is calibrated. For example, if your balance is not calibrated correctly, you can make very precise, repeated measurements, but the measurements will not represent the true value. Precision, on the otherhand, is usually determined by how careful the scientist is in making measurements. If you are careless and spill part of your sample on the way, your measurements in repeated experiments will not be precise even if your balance is accurate.
Practice Problems with Accuracy and Precision
Significant Figures
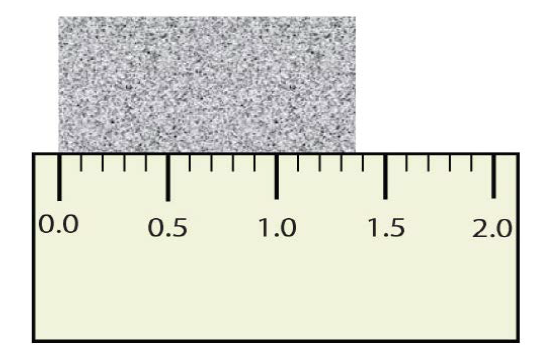
It is important to realize that values in scientific measurements are never 100% accurate. Our instruments only measure to a certain level of accuracy. Thus, we can pick different instruments to make a measurement based upon the level of accuracy we need for the experiment. Due to the inherent inaccuracy in any measured number we must keep track of the different levels of accuracy each number has with significant figures. Significant figures of a measured quantity are defined as all the digits known with certainty and the first uncertain, or estimated, digit. It makes no sense to report any digits after the first uncertain one, so it is the last digit reported in a measurement. Zeros are used when needed to place the significant figures in their correct positions. Thus, zeros may or may not be significant figures. Significant figures apply in the real world, as they allow us to quantify the accuracy of any type of measurement. To identify how many numbers in a measurement have significance, you can follow a discreet set of rules, shown below and to the right.
Figure 1.6: Measuring an Object to the Correct Number of Significant Figures.
How many digits should be shown in this measurement?
The correct answer is 3! The two that you know for sure + the estimated position…for this reading it would be close to 1.37
Exact Numbers
Exact numbers are numbers that are not measured by a scientific instrument. They are either used as definitions to define a concept or terminology, or they are made by counting the total of something present. An example of an exact number, would be the number of eggs in a carton or a defined unit such as there are 100 cm in 1 m. Exact numbers, such as the number of people in a room, DO NOT affect the number of significant figures in calculations made with measured values.
Quiz Yourself: More Practice with Significant Figures
Rules of Rounding
In scientific operations, the rules of rounding may be a little bit different than the ones you are used to using. Normal rounding rules suggest that if a number is below 5, it should be rounded down to the lower number, whereas if it is 5 or higher, it should be rounded up. However, note that 5 is right in the middle and causes a problem when using these conventional rounding rules. If you have a large dataset of numbers that you need to round, using this rounding rule will lead to bias in your dataset (i.e. 4/9th of the time you will be rounding down, and 5/9th of the time you will be rounding up). In a large dataset, this bias is unacceptable.
In Scientific Rounding, we typically use a rule called ‘Rounding to the Even.’ In this rounding system the rules are the same for a number below 5, you round down to the lower number, and for numbers above 5 you round up to the higher number. That means If the number you are rounding is 5 and it has any following numbers, all of the excess digits are dropped and the last retained digit is increased by one. However, if the number you are rounding is exactly 5 or 5 with only zeros following it, then you round to the even number. This helps to alleviate the sample bias that can occur when rounding large datasets.
Example 1:
Round 26.65 to three significant figures.
Solution:
When you look at the 4th digit, it is a 5 with no numbers following it. So now you must look at the 3rd digit. In this case it is even, so you would round to the even and the final answer would be 26.6
Example 2:
Round 26.652 to three significant figures.
Solution:
When you look at the 4th digit, it is a 5 with a number following it. In this case both the 5 and the 2 will be dropped and the remaining digit must be increased by one, so you would round the 6 up and the final answer would be 26.7
Example 3:
Round 26.7500 to three significant figures.
Solution:
When you look at the 4th digit, it is a 5 with only zeros following it. So now you must look at the 3rd digit. In this case it is odd, so you would round up to the even and the final answer would be 26.8
Calculations with Significant Figures
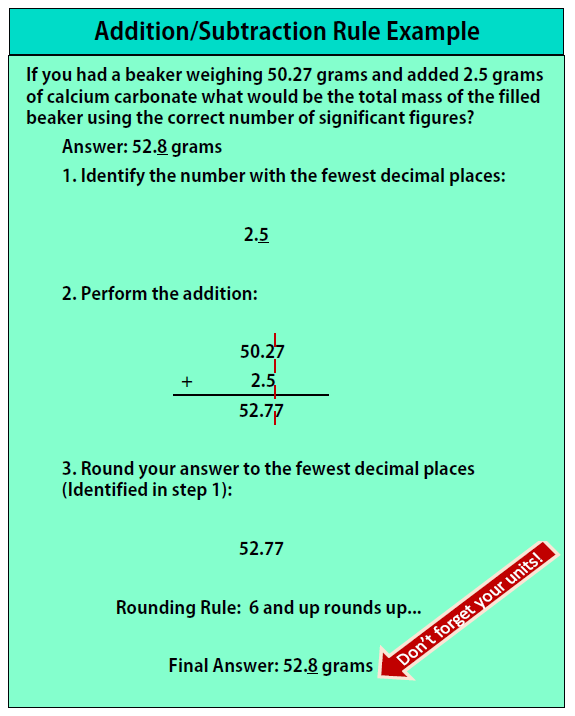
The first thing to realize before performing any calculations in science is that all measured numbers are only as good as the instrument used to measure them. Even with the best instrument available the measured number will never be 100% exact. Scientists use the “good enough” rule of precision, meaning that we accept an inherent amount of imprecision from every measurement we take as long as the final result is close enough to where we want it to be. This concept becomes dangerous when we begin to use these “good enough” numbers for any calculations, if we aren’t careful to keep track of our significant figures our numbers can quickly lose their “good enough” status. To protect their “good enough” numbers, the scientific community has set forth certain rules for performing any calculations; in this section we need only concern ourselves with two very important rules: the Addition/Subtraction rule, and the Multiplication/Division rule.
Addition/Subtraction Rule:
- Find the number with the least number of decimals and keep track of the number of decimal places
- Perform the addition/subtraction
- Round the final answer to the least number of decimals found in Step 1
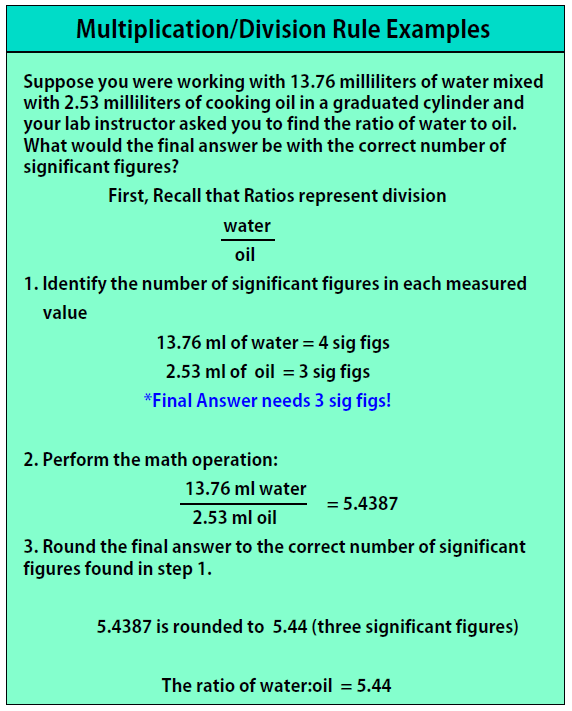
Multiplication/Division Rule:
- Count the number of significant figures in each number (keep track of the number of significant figures)
- Perform the multiplication/division
- Round your final answer to the lowest number of significant figures found in step 1
Calculating Complicated Problems:
- Using the order of operations, break the problem up into multiple steps
- Perform any addition/subtraction steps following the Addition/Subtraction rule (Do not round yet, just keep track of the correct number of decimals when finding the number of significant figures)
- Perform multiplication/division using the Multiplication/Division rule
- Round the final answer to the correct number of significant figures
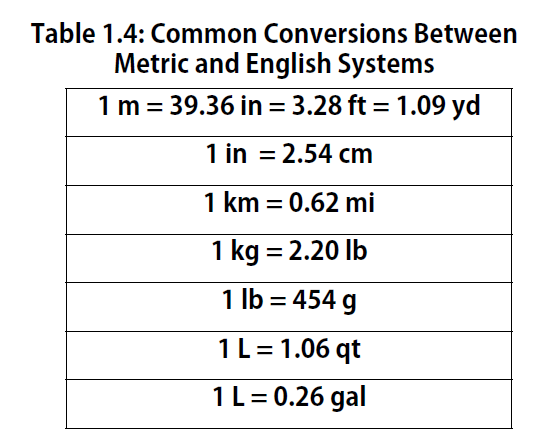
Conversions and the Importance of Units
The ability to convert from one unit to another is an important skill. For example, a nurse with 50 mg aspirin tablets who must administer 0.2 g of aspirin to a patient, needs to know that 0.2 g equals 200 mg, so that 4 tablets are needed. Fortunately, there is a simple way to convert from one unit to another.
Conversion Factors
If you learned the SI units and prefixes described in Section 1.4 Units of Measurement”, then you know that 1 cm is 1/100th of a meter or:
100 cm = 1 m
Suppose we divide both sides of the equation by 1 m (both the number and the unit; Note that it is critically important to always write out your units! This avoids confusion and mistakes when making conversions.):
As long as we perform the same operation on both sides of the equals sign, the expression remains an equality. Look at the right side of the equation; it now has the same quantity in the numerator (the top) as it has in the denominator (the bottom). Any fraction that has the same quantity in the numerator and the denominator has a value of 1:
We know that 100 cm is 1 m, so we have the same quantity on the top and the bottom of our fraction, although it is expressed in different units. A fraction that has equivalent quantities in the numerator and the denominator but expressed in different units is called a conversion factor
Note that conversion factors can be written with either term in the numerator or denominator, and used as appropriate for the problem that you want to solve. This is because, both terms are equal to 1
Here is a simple example. How many centimeters are there in 3.55 m? Perhaps you can determine the answer in your head. If there are 100 cm in every meter, then 3.55 m equals 355 cm. To solve the problem more formally with a conversion factor, we first write the quantity we are given, 3.55 m. Then we multiply this quantity by a conversion factor, which is the same as multiplying it by 1. We can write 1 as 100cm/1m and multiply:
Because m, the abbreviation for meters, occurs in both the numerator and the denominator of our expression, they cancel out. The final step is to perform the calculation that remains once the units have been canceled. Note that it is CRITICAL to retain the right units in the final answer or it will not make sense. A generalized description of this process is as follows:
quantity (old units) × conversion factor = quantity (new units)
You may be wondering why we use a seemingly complicated procedure for a straightforward conversion. In later studies, the conversion problems you will encounter will not always be so simple. If you can master the technique of applying conversion factors, you will be able to solve a large variety of problems.
In the previous example, we used the fraction 100 cm/1 m as a conversion factor. Does the conversion factor 1 m/100 cm also equal 1? Yes, it does; it has the same quantity in the numerator as in the denominator (except that they are flip-flopped). Why did we not use that conversion factor? If we had used the second conversion factor, the original unit would not have canceled, and the result would have been meaningless. Here is what we would have gotten:
INCORRECT USE OF CONVERSION FACTOR!!
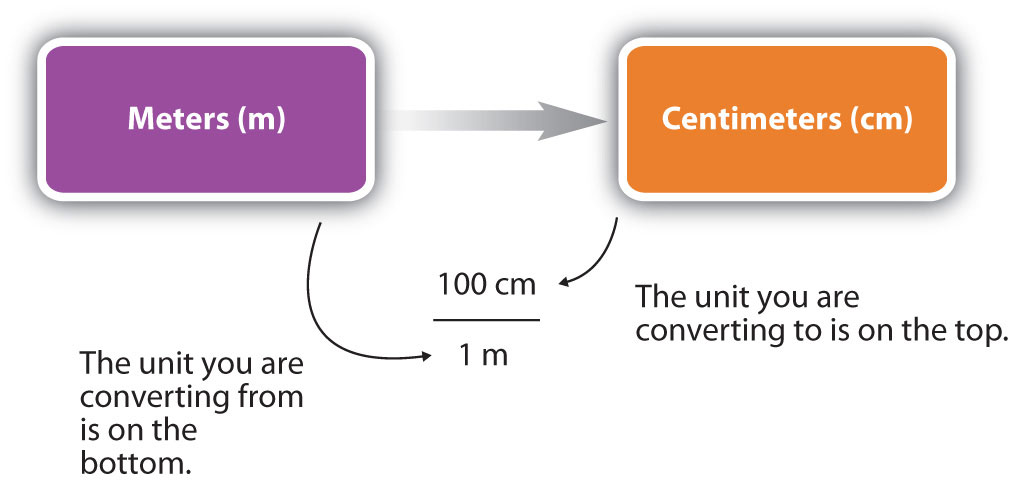
You can see that none of the units cancelled out. For the answer to be meaningful, we have to construct the conversion factor in a form that causes the original unit to cancel out. Figure 1.13 “A Concept Map for Conversions” shows a concept map for constructing a proper conversion.
Figure 1.13 A Concept Map for Conversions. This is how you construct a conversion factor to convert from one unit to another.
Chapter Summary
- Scientific notation is a useful way to represent very large and very small numbers. Note that when using scientific notation, that all numbers shown are significant figures.
- The International System of Units (SI) and the Metric System are the standard units of measurement used when making scientific measurements. These include direct measurements for mass (grams), length (meter), temperature (Celcius), time (second), and amount (mole) and the derived units for volume (liter), energy (joule), and density (mass/volume or g/mL).
- The validity of scientific experiments depend on both precision and accuracy. Precision is a measure of the repeatability of the measurements and is usually dependent on how carefully a researcher performs the experiment. Accuracy is a measure of the correctness of the collected or analyzed data and is usually dependent on how well the equipment is calibrated and working at the time of the measurements.
- Significant figures are used to indicate the accuracy of scientific measurements and are dependent on the equipment used to make the measurement. Significant figures in scientific measurements include the first estimated position of a measurement. No additional estimated positions are included.
- When representing a scientific value using significant figures, it may be necessary to round the number to the correct number of digits. For scientific rounding, we use the concept of rounding to the evens. For numbers below 5 always round down and for numbers larger than 5 always round up. However, if the number you are rounding is exactly 5 or 5 with only zeros following it, round the previous number to the even number. (i.e. 6.5 would be rounded down to 6, whereas 7.5 would be rounded up to 8)
- When making calculations, it is necessary to maintain the correct number of significant figures within your answer. The least number of decimal places is critical for finding significant figures after addition and subtraction functions, whereas the least number of significant figures within the starting numbers is important for multiplication and division functions. Recall that only measured numbers are used in the determination of significant figures. Exact numbers such as defined units and conversion factors are not used to determine significant figures. It is also important to only round a calculated number at the final step in the calculation.
- When making calculations, always include the appropriate units within your calculations and utilize appropriate conversion factors when required.
Chapter Homework:
Practice with Conversions, the Factor Label Method, and Density
References:
Chapter 1 materials have been adapted and modified from the following creative commons resources unless otherwise noted:
1. Anonymous. (2012) Introduction to Chemistry: General, Organic, and Biological (V1.0). Published under Creative Commons by-nc-sa 3.0. Available at: http://2012books.lardbucket.org/books/introduction-to-chemistry-general-organic-and-biological/index.html
2. Poulsen, T. (2010) Introduction to Chemistry. Published under Creative Commons by-nc-sa 3.0. Available at: http://openedgroup.org/books/Chemistry.pdf
3. OpenStax (2015) Atoms, Isotopes, Ions, and Molecules: The Building Blocks. OpenStax CNX.Available at: http://cnx.org/contents/be8818d0-2dba-4bf3-8