Home » Student Resources » Online Chemistry Textbooks » Foundations in General, Organic, and Biological Chemistry » Chapter 6 – Quantities in Chemical Reactions
MenuChapter 6 – Quantities in Chemical Reactions
Chapter 6 – Quantities in Chemical Reactions
This text is published under creative commons licensing, for referencing and adaptation, please click here.
6.1: Chapter Introduction
6.2: The Mole
6.3: Atomic and Molar Mass
6.4: Mole-Mass Conversions
6.5: Mole-Mole Relationships in Chemical Reactions
6.6: Mole-Mass and Mass-Mass Problems
6.7: Chapter Summary
6.8: References
6.1: Chapter Introduction
So far, we have talked about chemical reactions in terms of individual atoms and molecules. Although this works, most of the reactions occurring around us involve much larger amounts of chemicals. Even a tiny sample of a substance will contain millions, billions, or a hundred billion billions of atoms and molecules. How do we compare amounts of substances to each other in chemical terms when it is so difficult to count to a hundred billion billion?
Actually, there are ways to do this, which we will explore in this chapter. In doing so, we will increase our understanding of stoichiometry, which is the study of the numerical relationships between the reactants and the products in a balanced chemical reaction.
(Back to the Top)
6.2: The Mole
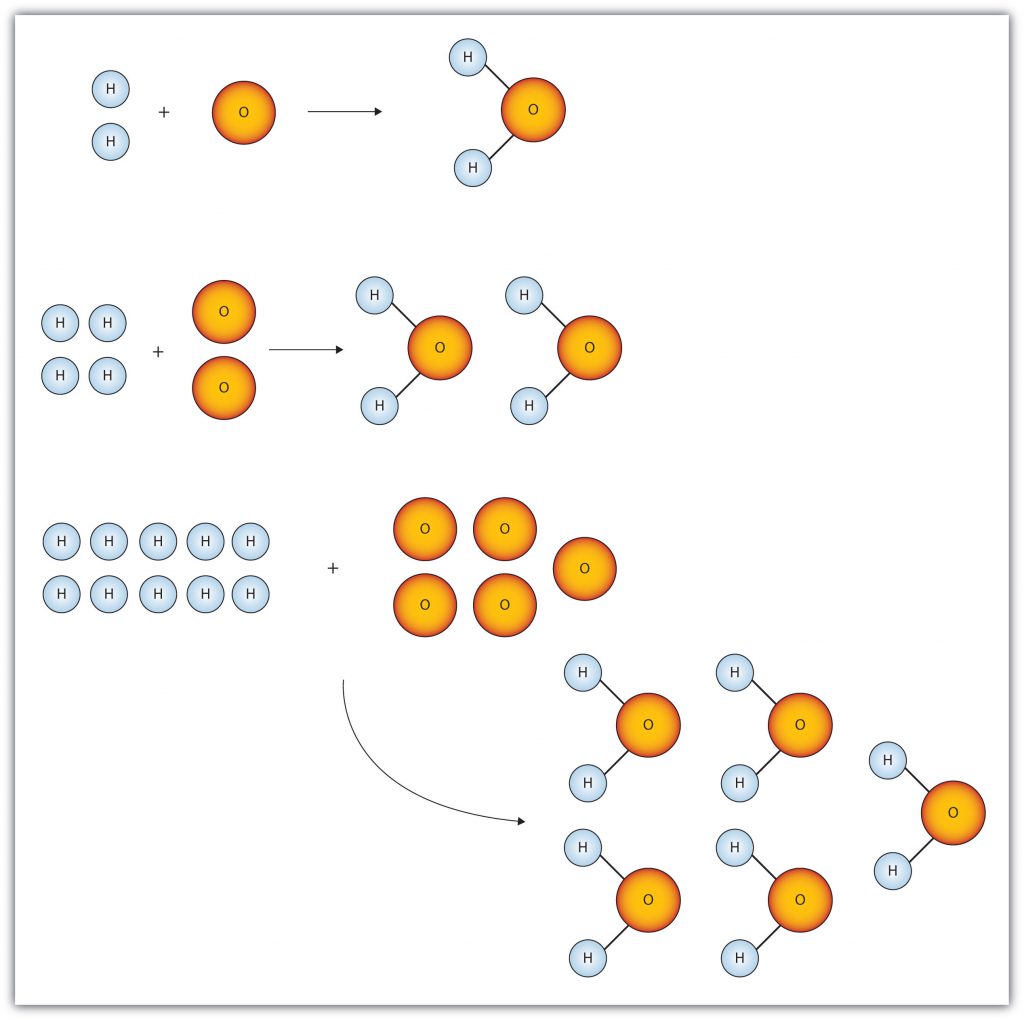
Figure 6.1 “Water Molecules” shows that we need 2 hydrogen atoms and 1 oxygen atom to make 1 water molecule. If we want to make 2 water molecules, we will need 4 hydrogen atoms and 2 oxygen atoms. If we want to make 5 molecules of water, we need 10 hydrogen atoms and 5 oxygen atoms. The ratio of atoms we will need to make any number of water molecules is the same: 2 hydrogen atoms to 1 oxygen atom.
Figure 6.1 Water Molecules: The ratio of hydrogen atoms to oxygen atoms used to make water molecules is always 2:1, no matter how many water molecules are being made.
One problem we have, however, is that it is extremely difficult, if not impossible, to organize atoms one at a time. As stated in the introduction, we deal with billions of atoms at a time. How can we keep track of so many atoms (and molecules) at a time? We do it by using mass rather than by counting individual atoms.
A hydrogen atom has a mass of approximately 1 u. An oxygen atom has a mass of approximately 16 u. The ratio of the mass of an oxygen atom to the mass of a hydrogen atom is therefore approximately 16:1.
If we have 2 atoms of each element, the ratio of their masses is approximately 32:2, which reduces to 16:1—the same ratio. If we have 12 atoms of each element, the ratio of their total masses is approximately (12 × 16):(12 × 1), or 192:12, which also reduces to 16:1. If we have 100 atoms of each element, the ratio of the masses is approximately 1,600:100, which again reduces to 16:1. As long as we have equal numbers of hydrogen and oxygen atoms, the ratio of the masses will always be 16:1.
The same consistency is seen when ratios of the masses of other elements are compared. For example, the ratio of the masses of silicon atoms to equal numbers of hydrogen atoms is always approximately 28:1, while the ratio of the masses of calcium atoms to equal numbers of lithium atoms is approximately 40:7.
So we have established that the masses of atoms are constant with respect to each other, as long as we have the same number of each type of atom. Consider a more macroscopic example. If a sample contains 40 g of Ca, this sample has the same number of atoms as there are in a sample of 7 g of Li. What we need, then, is a number that represents a convenient quantity of atoms so we can relate macroscopic quantities of substances. Clearly even 12 atoms are too few because atoms themselves are so small. We need a number that represents billions and billions of atoms.
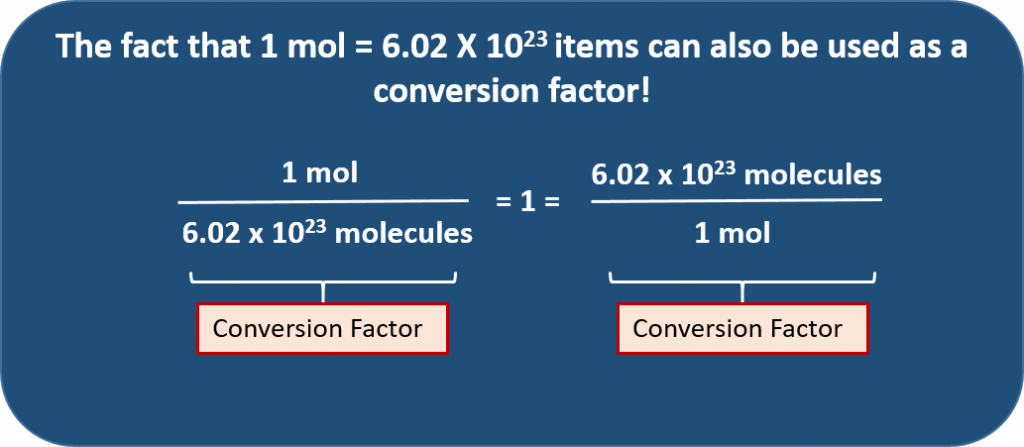
A mole is defined as 6.02 × 1023 items and thus, it is used by chemists to represent a large number of atoms or molecules. Just as a dozen implies 12 things, a mole (abbreviated mol) represents 6.02 × 1023 things. The number 6.02 × 1023, called Avogadro’s number, after the 19th-century chemist Amedeo Avogadro, is the number we use in chemistry to represent macroscopic amounts of atoms and molecules. Thus, if we have 6.02 × 1023 Oxygen atoms, we say we have 1 mole of Oxygen atoms. If we have 2 mol of Na atoms, we have 2 × (6.02 × 1023) Na atoms, or 1.2044 × 1024 Na atoms. Similarly, if we have 0.5 mol of benzene (C6H6) molecules, we have 0.5 × (6.02 × 1023) C6H6 molecules, or 3.011 × 1023 C6H6 molecules.
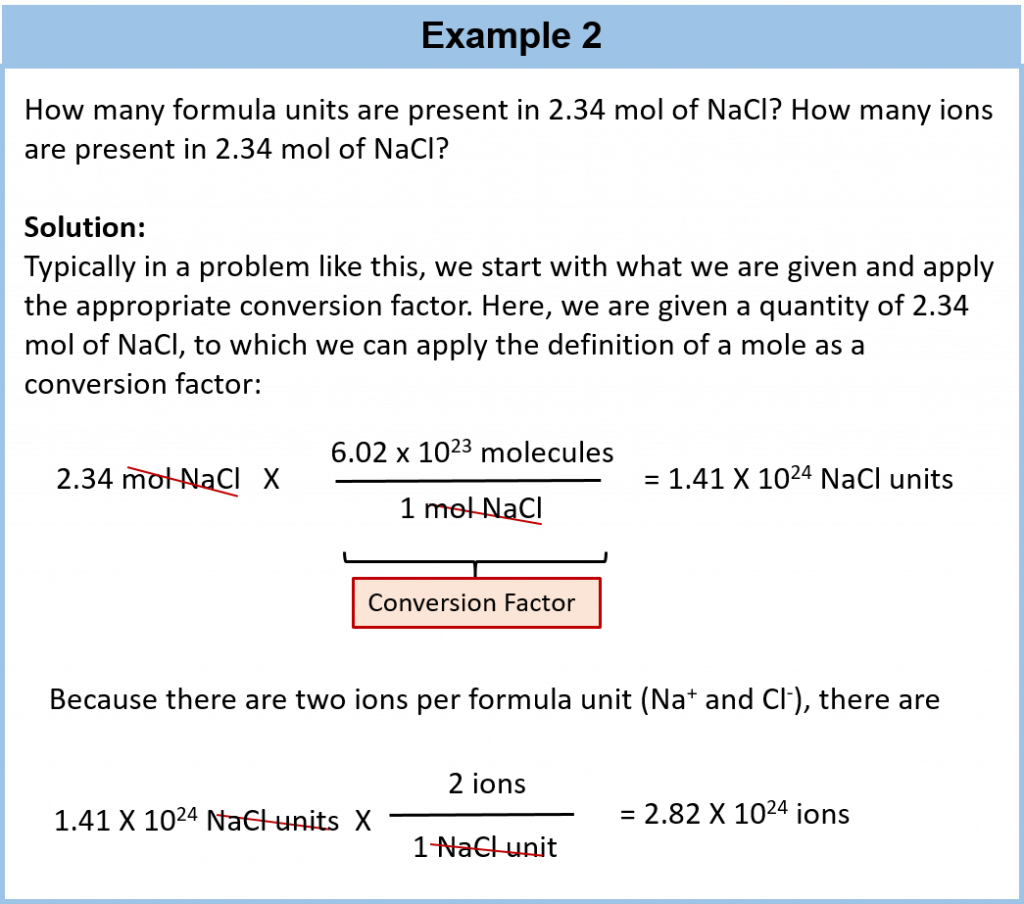
Notice that we are applying the mole unit to different types of chemical entities. In these examples, we cited moles of atoms and moles of molecules. The word mole represents a number of things—6.02 × 1023 of them—but does not by itself specify what “they” are. They can be atoms, formula units (of ionic compounds), or molecules. That information still needs to be specified.
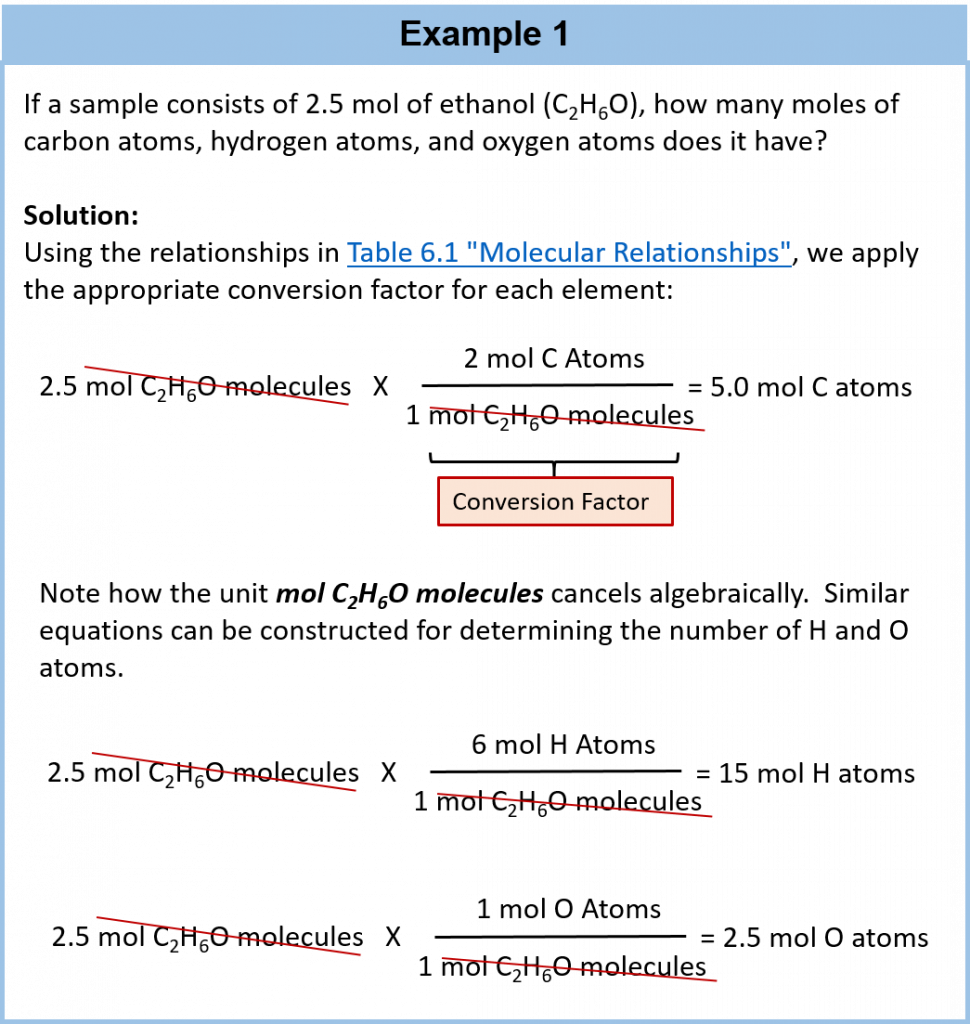
Because 1 H2 molecule contains 2 H atoms, 1 mol of H2 molecules (6.02 × 1023 molecules) has 2 mol of H atoms. Using formulas to indicate how many atoms of each element we have in a substance, we can relate the number of moles of molecules to the number of moles of atoms. For example, in 1 mol of ethanol (C2H6O), we can construct the following relationships (Table 6.1 “Molecular Relationships”):
Table 6.1: Molecular Relationships
The following example illustrates how we can use these relationships as conversion factors.
(Back to the Top)
6.3: Atomic and Molar Mass
Now that we have introduced the mole and practiced using it as a conversion factor, we ask the obvious question: why is the mole that particular number of things? Why is it 6.022 × 1023 and not 1 × 1023 or even 1 × 1020?
The number in a mole, Avogadro’s number, is related to the relative sizes of the atomic mass unit and gram mass units. Whereas one hydrogen atom has a mass of approximately 1 amu, 1 mol of H atoms has a mass of approximately 1 gram. And whereas one sodium atom has an approximate mass of 23 amu, 1 mol of Na atoms has an approximate mass of 23 grams.
One mole of a substance has the same mass in grams that one atom or molecule has in atomic mass units. The numbers in the periodic table that we identified as the atomic masses of the atoms not only tell us the mass of one atom in atomic mass units, but also tell us the mass of 1 mole of atoms in grams! This is because all atoms are made up of the same parts (protons, neutrons, and electrons) with the protons and neutrons having nearly identical masses. Electrons, since they are so light, are negligent in their contribution to atomic mass, even in the largest atoms. Thus, an atomic or molecular mass is indicative of how many atoms or molecules are present. Thus, an important three way relationship is formed: 1 mol = atomic or molecular mass in grams = 6.02 X 1023 atoms or molecules. This effectively gives us a way to count molecules in the laboratory using a common balance! Note that in chemical equations and calculations that mole concentrations are abbreviated as mol.
Recall that, the mass of an ionic compound (referred to as the formula mass) or a covalent molecule (referred to as the molecular mass)—is simply the sum of the masses of its atoms. To calculate formula or molecular masses, it is important that you keep track of the number of atoms of each element in the molecular formula to obtain the correct molecular mass.
For Example:
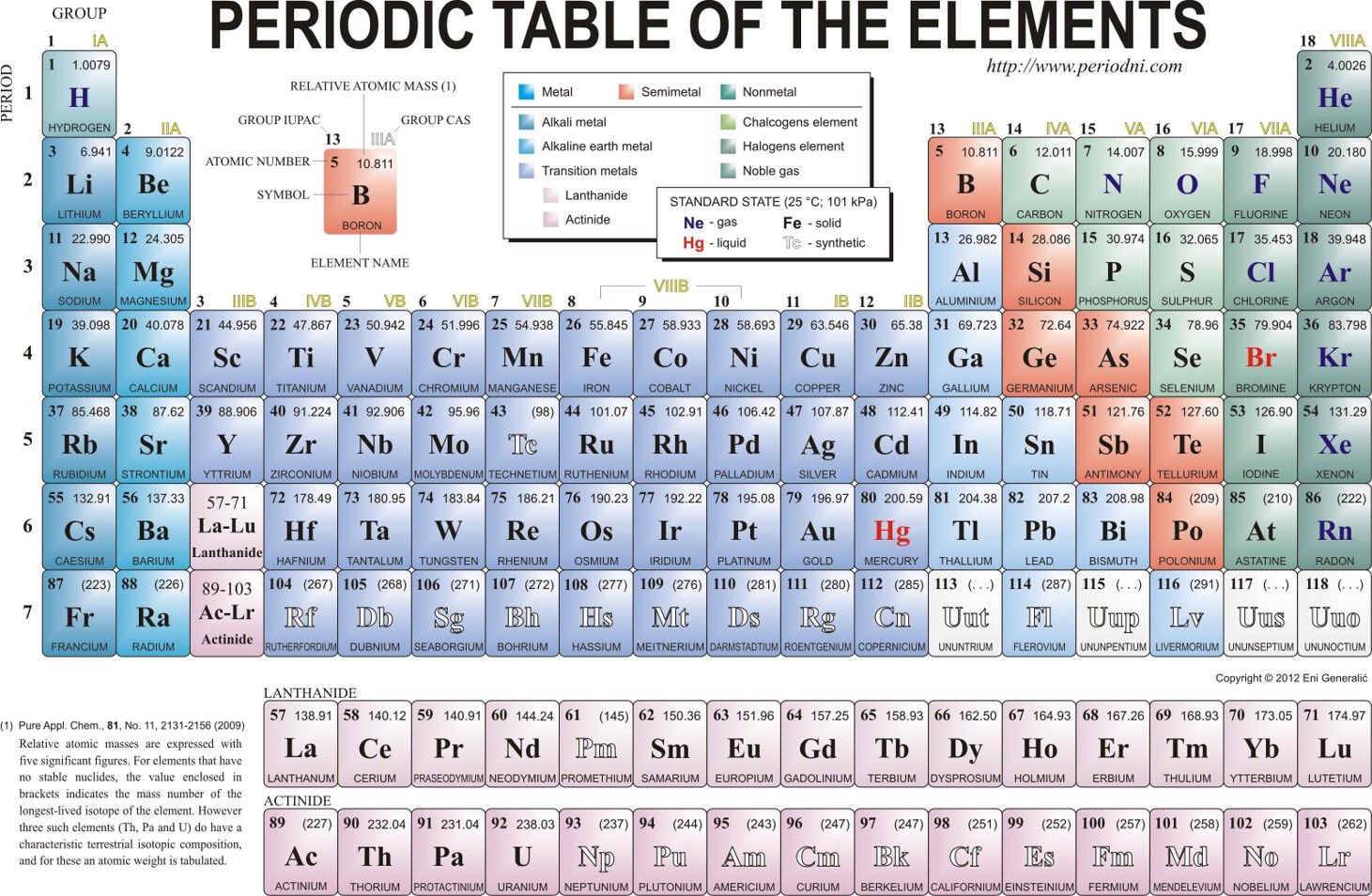
A molecule of NaCl contains 1 Na+ and 1 Cl–. Thus, we can the formula mass of this compound by adding together the atomic masses of sodium and chlorine, as found on the periodic table (Figure 6.1).
Figure 6.1 Periodic Table of the Elements
For a larger molecule, like glucose (C6H12O6), that has multiple atoms of the same type, simply multiply the atomic mass of each atom by the number of atoms present, and then add up all the atomic masses to get the final molecular mass.
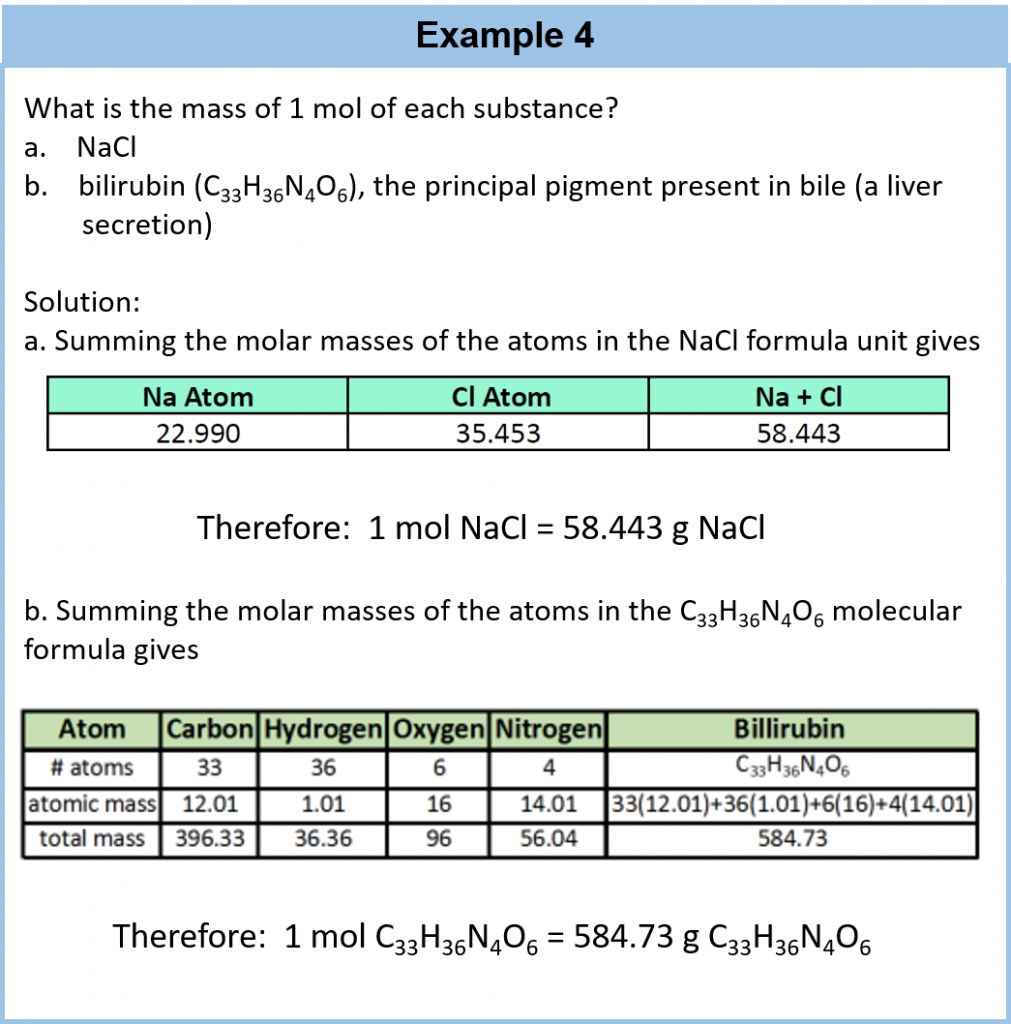
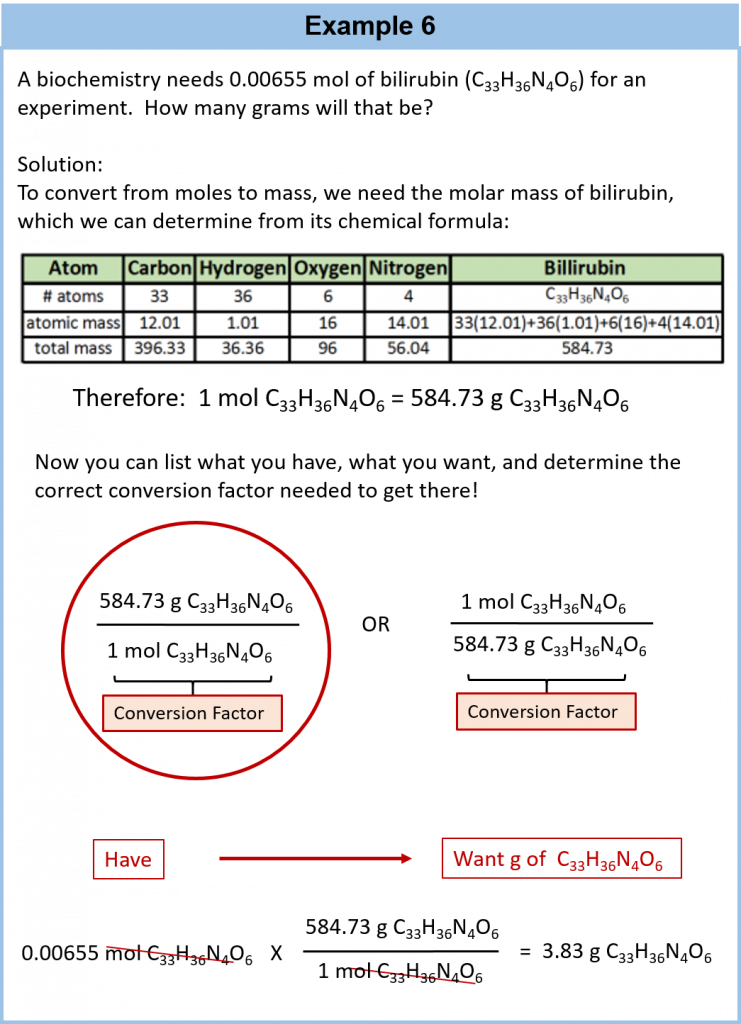
The mole concept can be extended to masses of formula units and molecules as well. The mass of 1 mol of molecules (or formula units) in grams is numerically equivalent to the mass of one molecule (or formula unit) in atomic mass units. For example, a single molecule of O2 has a mass of 32.00 u (the sum of 2 oxygen atoms), and 1 mol of O2 molecules has a mass of 32.00 g. As with atomic mass unit–based masses, to obtain the mass of 1 mol of a substance, we simply sum the masses of the individual atoms in the formula of that substance. The mass of 1 mol of a substance is referred to as its molar mass, whether the substance is an element, an ionic compound, or a covalent compound.
Figure 6.2: The Amazing Mole. The major mole conversion factors are shown.
The relationship of the mole quantities to gram conversion factors listed above in Figure 6.2 are notably some of the most useful equations in all of chemistry. They make it possible to set up chemical reactions in a safe and efficient manner and they have tremendous impact on the economics of many industrial and manufacturing processes and the production of medicine. If you are a serious student of chemistry, I would recommend printing out table 6.2 and keeping a copy in your notebook. It will be extremely useful in setting up a multitude of word problems and is functionally useful in the laboratory.
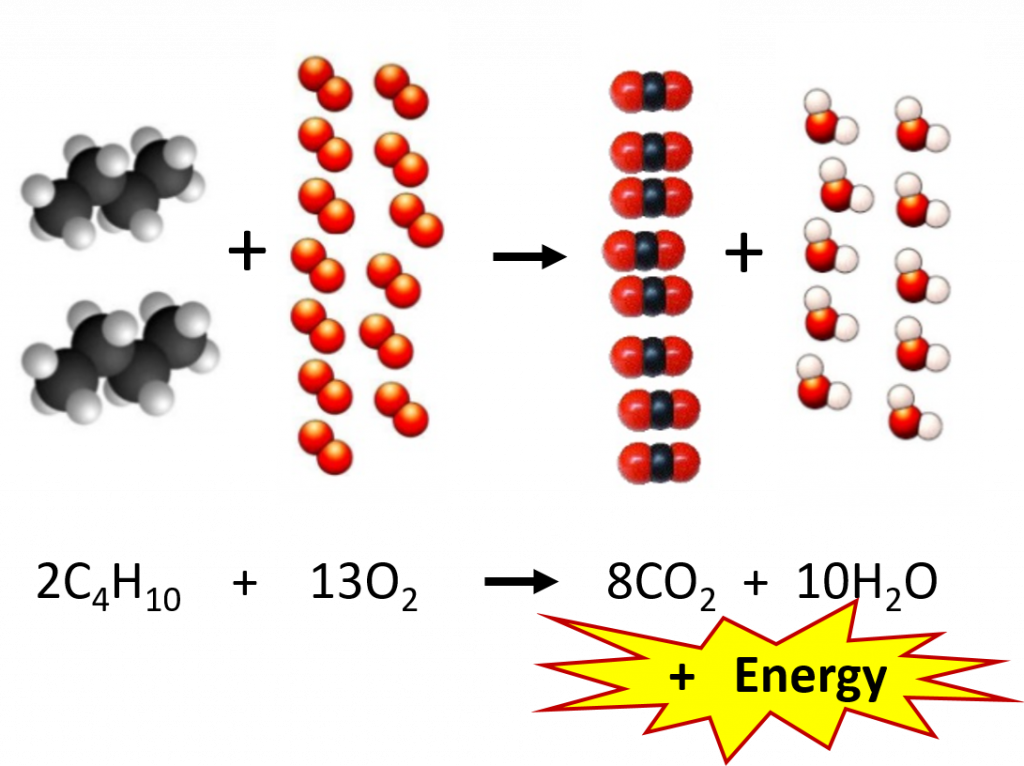
So why is the relationship between the mole and compound mass so important? In the microscopic world, chemical equations are set up on the scale of molecules. Figure 6.3 depicts a typical chemical reaction. As we learned in Chapter 5, the coefficients in front of each compound represent the number of molecules needed for the reaction to proceed. In this combustion reaction, 2 molecules of butane (C4H10) react with 13 molecules of oxygen to produce 8 molecules of carbon dioxide and 10 molecules of water. In the lab, however, chemists are unable to count out molecules and place them in a reaction flask. Molecules are way too small to be seen by the naked eye and there is no equipment available that is capable of sorting and counting molecules in this way. Mass, on the other hand, can easily be measured using a balance. Thus, the relationship of mass to the number of molecules present becomes a very important conversion. Since the mole represents a fixed number of molecules (6.02 X 1023), the coefficients used in chemical reactions can also be thought of as molar ratios. Instead of reading our equation in terms of molecules, we can read it in terms of moles. At the macroscopic level, the reaction below reads: 2 moles of butane (C4H10) react with 13 moles of oxygen to produce 8 moles of carbon dioxide and 10 moles of water.
Figure 6.3 Combustion Reaction of Butane. The example shows the molecular ratios of the substrates and products of the reaction.
6.4 Mole-Mass Conversions
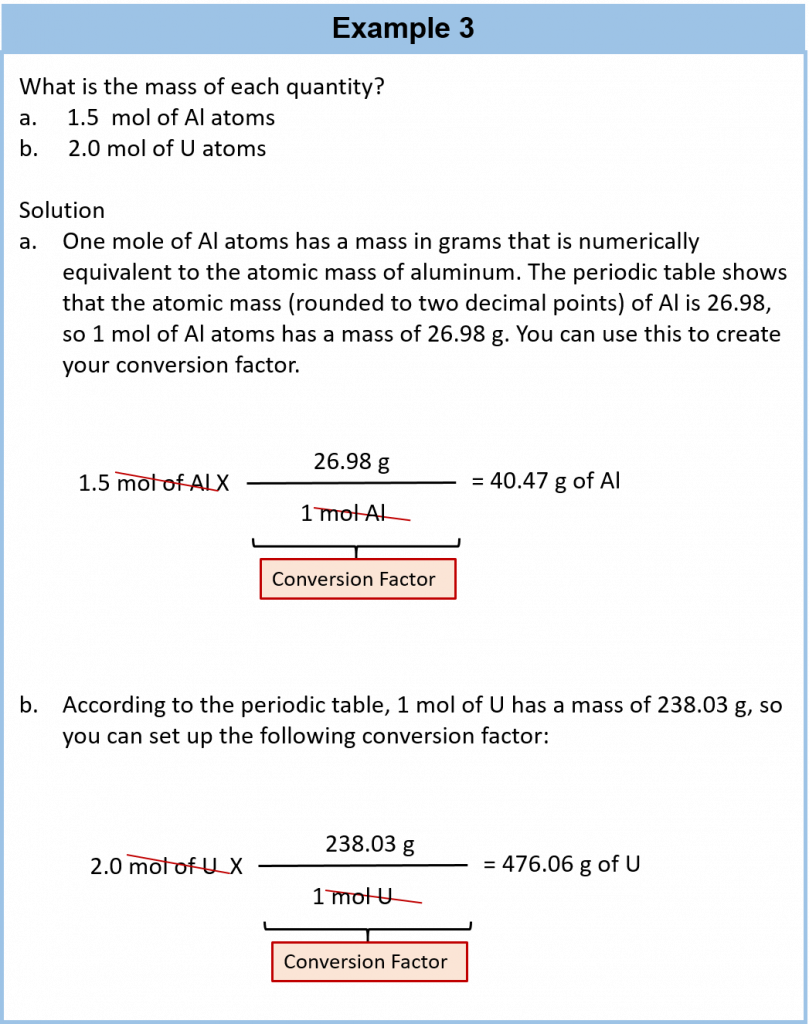
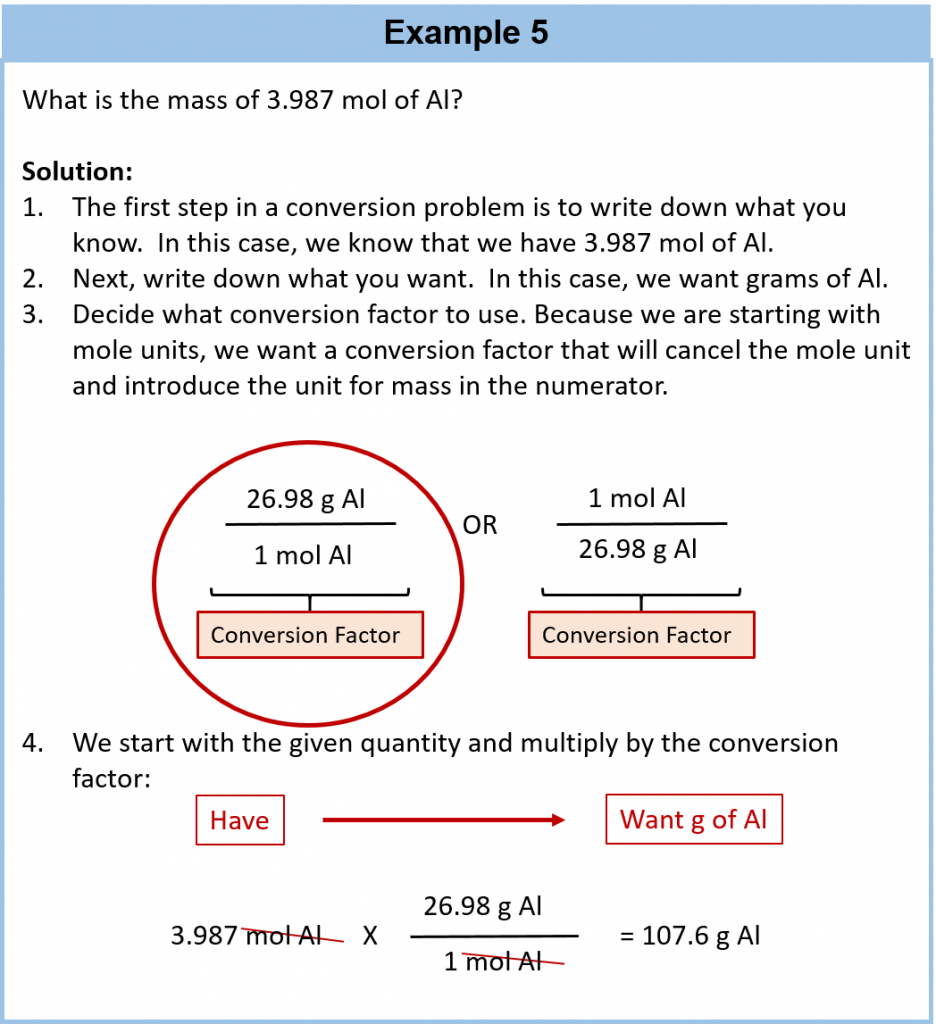
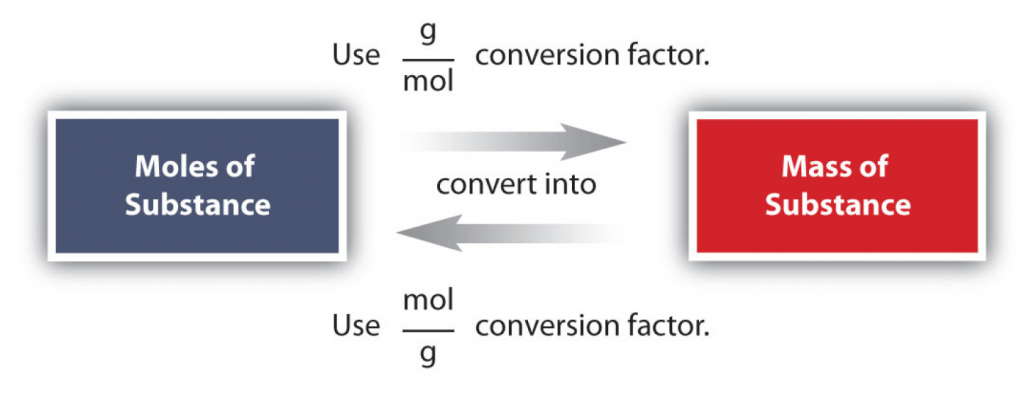
The simplest type of manipulation using molar mass as a conversion factor is a mole-mass conversion (or its reverse, a mass-mole conversion), as shown in Figure 6.2. In such a conversion, we use the molar mass of a substance as a conversion factor to convert mole units into mass units (or, conversely, mass units into mole units).
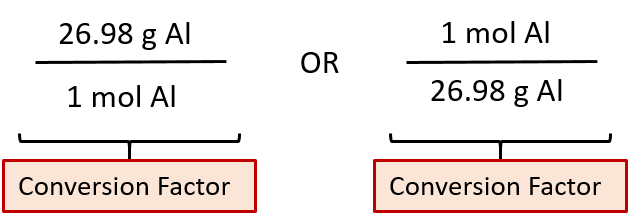
We established that 1 mol of Al has a mass of 26.98 g (Example 3 in Section 6.3). Stated mathematically,
1 mol Al = 26.98 g Al
We can divide both sides of this expression by either side to get one of two possible conversion factors:
The first conversion factor can be used to convert from mass to moles, and the second converts from moles to mass. Both can be used to solve problems.
Conversions like this are possible for any substance, as long as the proper atomic mass, formula mass, or molar mass is known (or can be determined) and expressed in grams per mole. Figure 6.4 is a chart for determining what conversion factor is needed, and Figure 6.5 is a flow diagram for the steps needed to perform a conversion. Note that it takes one mathematical step to convert from moles to mass or from mass to moles.
To Your Health: Minerals
For our bodies to function properly, we need to ingest certain substances from our diets. Among our dietary needs are minerals, the non-carbon elements, our body uses for a variety of functions, such developing bone or ensuring proper nerve transmission.
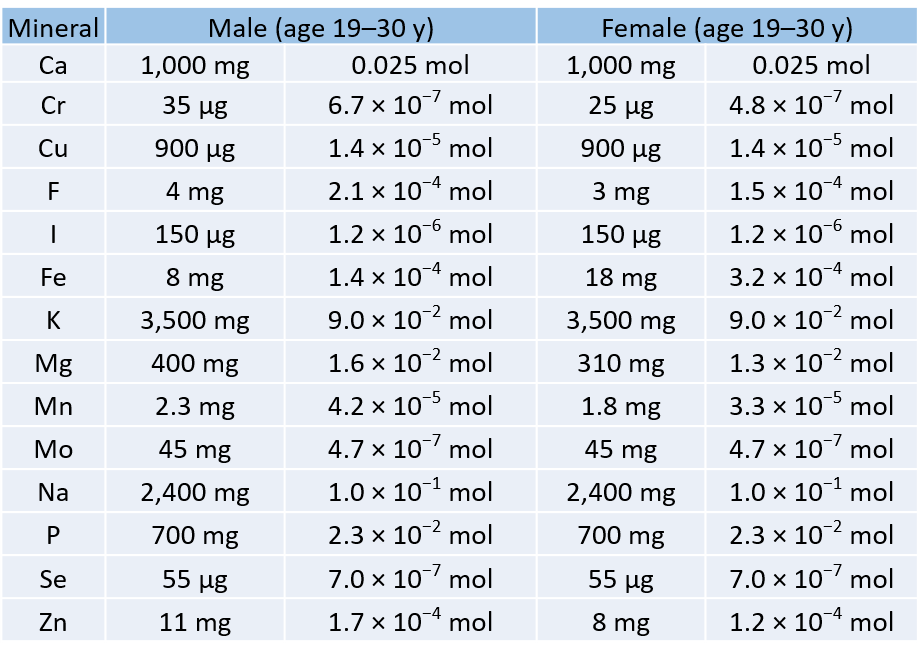
The US Department of Agriculture has established some recommendations for the daily intake (RDIs) of various minerals. The accompanying table lists the RDIs for minerals, both in mass and moles, assuming a 2,000-calorie daily diet.
Table 6.2: Recommended Daily Intake (RDIs) of Mineral Supplements
Table 6.2 illustrates several things. First, the needs of men and women for some minerals are different. The extreme case is for iron; women need over twice as much as men do. In all other cases where there is a different RDI, men need more than women.
Second, the amounts of the various minerals needed on a daily basis vary widely—both on a mass scale and a molar scale. The average person needs 0.1 mol of Na a day, which is about 2.5 g. On the other hand, a person needs only about 25–35 µg of Cr per day, which is under one millionth of a mole. As small as this amount is, a deficiency of chromium in the diet can lead to diabetes-like symptoms or neurological problems, especially in the extremities (hands and feet). For some minerals, the body does not require much to keep itself operating properly.
Although a properly balanced diet will provide all the necessary minerals, some people take dietary supplements. However, too much of a good thing, even minerals, is not good. Exposure to too much chromium, for example, causes a skin irritation, and certain forms of chromium are known to cause cancer (as presented in the movie Erin Brockovich).
(Back to the Top)
6.5 Mole-Mole Relationships in Chemical Reactions
In this section you will learn how to use a balanced chemical reaction to determine molar relationships between the substances.
In Chapter 5, you learned to balance chemical equations by comparing the numbers of each type of atom in the reactants and products. The coefficients in front of the chemical formulas represent the numbers of molecules or formula units (depending on the type of substance). Here, we will extend the meaning of the coefficients in a chemical equation.
Consider the simple chemical equation
2H2 + O2 → 2H2O
The convention for writing balanced chemical equations is to use the lowest whole-number ratio for the coefficients. However, the equation is balanced as long as the coefficients are in a 2:1:2 ratio. For example, this equation is also balanced if we write it as
4H2 + 2O2 → 4H2O
The ratio of the coefficients is 4:2:4, which reduces to 2:1:2. The equation is also balanced if we were to write it as
22H2 + 11O2 → 22H2O
because 22:11:22 also reduces to 2:1:2.
Suppose we want to use larger numbers. Consider the following coefficients:
12.044 × 1023 H2 + 6.022 × 1023 O2 → 12.044 × 1023 H2O
These coefficients also have the ratio 2:1:2 (check it and see), so this equation is balanced. But 6.022 × 1023 is 1 mol, while 12.044 × 1023 is 2 mol (and the number is written that way to make this more obvious), so we can simplify this version of the equation by writing it as
2 mol H2 + 1 mol O2 → 2 mol H2O
We can leave out the word mol and not write the 1 coefficient (as is our habit), so the final form of the equation, still balanced, is
2H2 + O2 → 2H2O
Now we interpret the coefficients as referring to molar amounts, not individual molecules. The lesson? Balanced chemical equations are balanced not only at the molecular level but also in terms of molar amounts of reactants and products. Thus, we can read this reaction as “two moles of hydrogen react with one mole of oxygen to produce two moles of water.”
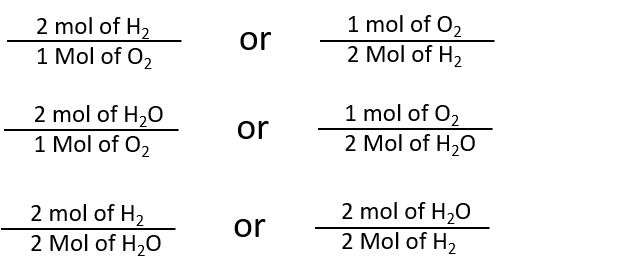
By the same token, the ratios we constructed in Chapter 5, can also be constructed in terms of moles rather than molecules. For the reaction in which hydrogen and oxygen combine to make water, for example, we can construct the following ratios:
We can use these ratios to determine what amount of a substance, in moles, will react with or produce a given number of moles of a different substance. The study of the numerical relationships between the reactants and the products in balanced chemical reactions is called stoichiometry.
6.6 Mole-Mass and Mass-Mass Problems
In this section you will learn to convert from mass or moles of one substance to mass or moles of another substance in a chemical reaction.
We have established that a balanced chemical equation is balanced in terms of moles as well as atoms or molecules. We have used balanced equations to set up ratios, now in terms of moles of materials, that we can use as conversion factors to answer stoichiometric questions, such as how many moles of substance A react with so many moles of reactant B. We can extend this technique even further. Recall that we can relate a molar amount to a mass amount using molar mass. We can use that ability to answer stoichiometry questions in terms of the masses of a particular substance, in addition to moles. We do this using the following sequence:
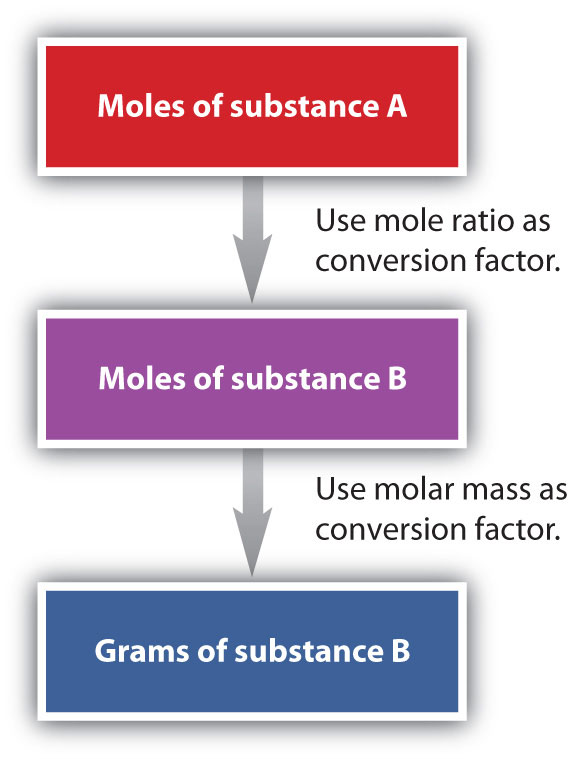
Figure 6.6: Flowchart for Calculating Mole to Mass Conversions using Chemical Equations.
Collectively, these conversions are called mole-mass calculations.
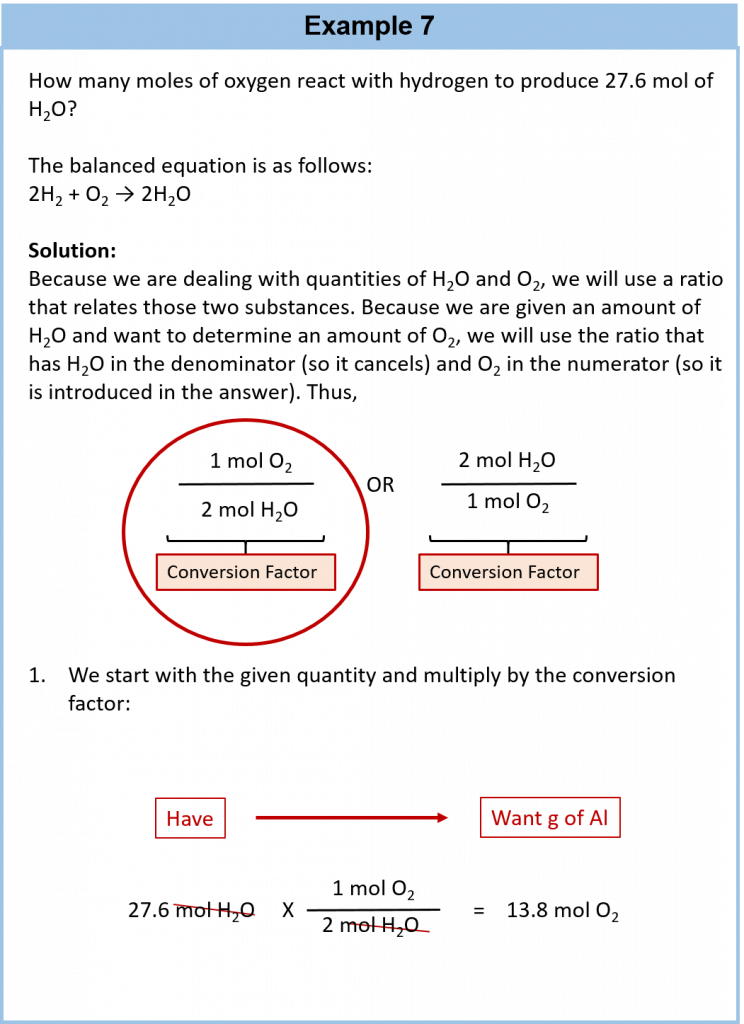
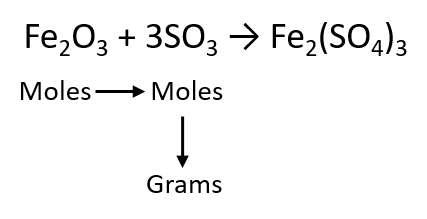
As an example, consider the balanced chemical equation
Fe2O3 + 3SO3 → Fe2(SO4)3
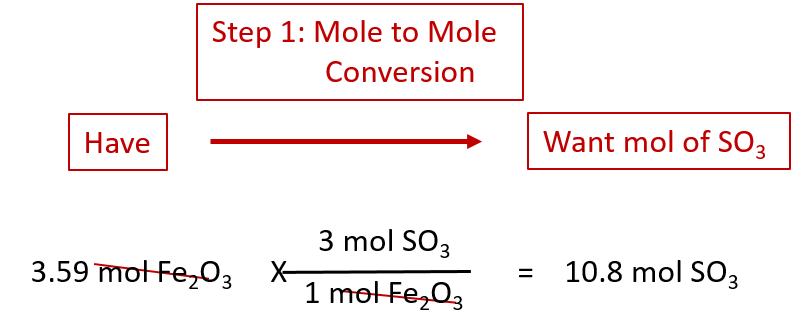
If we have 3.59 mol of Fe2O3, how many grams of SO3 can react with it? Using the mole-mass calculation sequence, we can determine the required mass of SO3 in two steps. First, we construct the appropriate molar ratio, determined from the balanced chemical equation, to calculate the number of moles of SO3 needed. Then using the molar mass of SO3 as a conversion factor, we determine the mass that this number of moles of SO3 has. Graphically, it is represented in these two steps:
The first step resembles the exercises we did in Section 6.4 “Mole-Mole Relationships in Chemical Reactions”. As usual, we start with the quantity we were given:
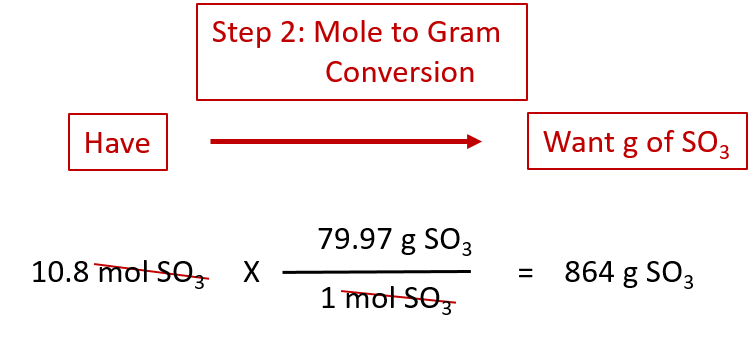
The mol Fe2O3 units cancel, leaving mol SO3 unit. Now, we take this answer and convert it to grams of SO3, using the molar mass of SO3 as the conversion factor:
Our final answer is expressed to three significant figures. Thus, in a two-step process, we find that 862 g of SO3 will react with 3.59 mol of Fe2O3. Many problems of this type can be answered in this manner.
The same two-step problem can also be worked out in a single line, rather than as two separate steps, as follows:
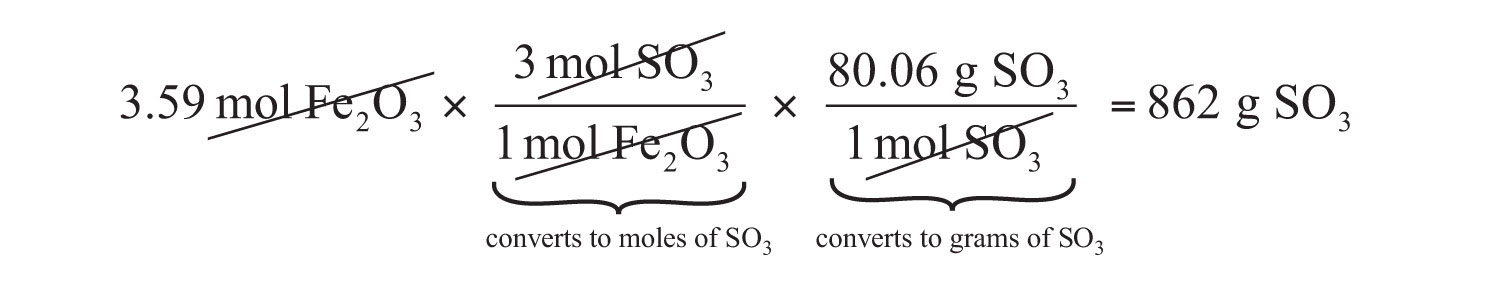
We get exactly the same answer when combining all the math steps together as we do when we calculate one step at a time.

It is a small step from mole-mass calculations to mass-mass calculations. If we start with a known mass of one substance in a chemical reaction (instead of a known number of moles), we can calculate the corresponding masses of other substances in the reaction. The first step in this case is to convert the known mass into moles, using the substance’s molar mass as the conversion factor. Then—and only then—we use the balanced chemical equation to construct a conversion factor to convert that quantity to moles of another substance, which in turn can be converted to a corresponding mass. Sequentially, the process is as follows:
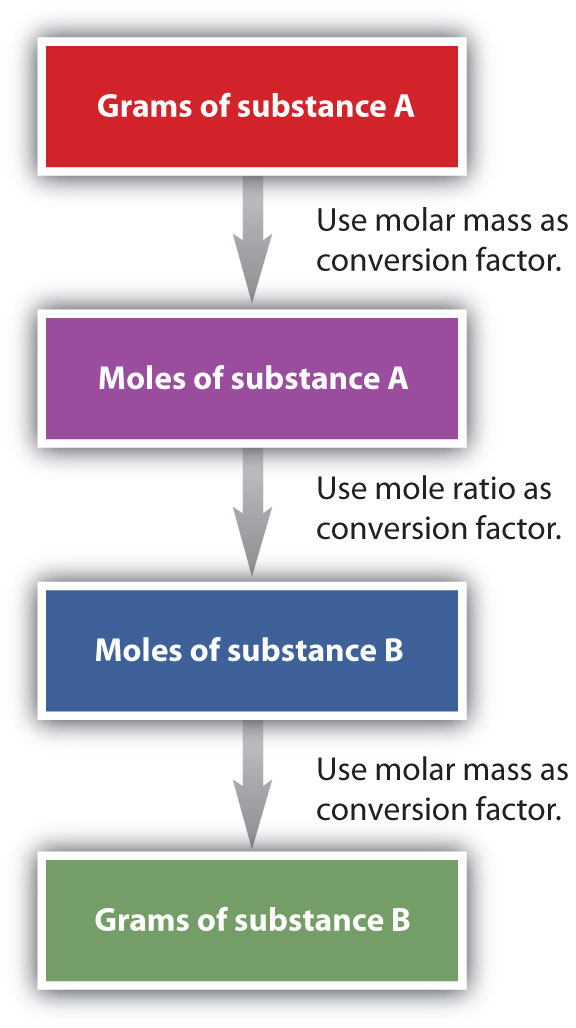
This three-part process can be carried out in three discrete steps or combined into a single calculation that contains three conversion factors. The following example illustrates both techniques.

To Your Health: The Synthesis of Taxol
Taxol is a powerful anticancer drug that was originally extracted from the Pacific yew tree (Taxus brevifolia). As you can see from the accompanying figure, taxol is a very complicated molecule, with a molecular formula of C47H51NO14. Isolating taxol from its natural source presents certain challenges, mainly that the Pacific yew is a slow-growing tree, and the equivalent of six trees must be harvested to provide enough taxol to treat a single patient. Although related species of yew trees also produce taxol in small amounts, there is significant interest in synthesizing this complex molecule in the laboratory.
After a 20-year effort, two research groups announced the complete laboratory synthesis of taxol in 1994. However, each synthesis required over 30 separate chemical reactions, with an overall efficiency of less than 0.05%. To put this in perspective, to obtain a single 300 mg dose of taxol, you would have to begin with 600 g of starting material. To treat the 26,000 women who are diagnosed with ovarian cancer each year with one dose, almost 16,000 kg (over 17 tons) of starting material must be converted to taxol. Taxol is also used to treat breast cancer, with which 200,000 women in the United States are diagnosed every year. This only increases the amount of starting material needed.
Clearly, there is intense interest in increasing the overall efficiency of the taxol synthesis. An improved synthesis not only will be easier but also will produce less waste materials, which will allow more people to take advantage of this potentially life-saving drug.
Figure 6.8 The Structure of the Cancer Drug Taxol. Because of the complexity of the molecule, hydrogen atoms are not shown, but they are present on every atom to give the atom the correct number of covalent bonds (four bonds for each carbon atom).
Key Takeaway
- A balanced chemical equation can be used to relate masses or moles of different substances in a reaction.
Exercises
-
Given the following unbalanced chemical equation,
H3PO4 + NaOH → H2O + Na3PO4
what mass of H2O is produced by the reaction of 2.35 mol of H3PO4?
-
Given the following unbalanced chemical equation,
C2H6 + Br2 → C2H4Br2 + HBr
what mass of HBr is produced if 0.884 mol of C2H6 is reacted?
-
Certain fats are used to make soap, the first step being to react the fat with water to make glycerol (also known as glycerin) and compounds called fatty acids. One example is as follows:
C3H5(OOC(CH2)14CH3)3a fat+3H2O→C3H5(OH)3glycerol+3CH3(CH2)14COOHfatty acid
-
How many moles of glycerol can be made from the reaction of 1,000.0 g of C3H5(OOC(CH2)14CH3)3?
-
Photosynthesis in plants leads to the general overall reaction for producing glucose (C6H12O6):
6CO2 + 6H2O → C6H12O6 + 6O2
How many moles of glucose can be made from the reaction of 544 g of CO2?
-
Precipitation reactions, in which a solid (called a precipitate) is a product, are commonly used to remove certain ions from solution. One such reaction is as follows:
Ba(NO3)2(aq) + Na2SO4(aq) → BaSO4(s) + 2NaNO3(aq)
How many grams of Na2SO4 are needed to precipitate all the barium ions produced by 43.9 g of Ba(NO3)2?
-
Nitroglycerin [C3H5(ONO2)3] is made by reacting nitric acid (HNO3) with glycerol [C3H5(OH)3] according to this reaction:
C3H5(OH)3 + 3HNO3 → C3H5(ONO2)3 + 3H2O
If 87.4 g of HNO3 are reacted with excess glycerol, what mass of nitroglycerin can be made?
-
Antacids are bases that neutralize acids in the digestive tract. Magnesium hydroxide [Mg(OH)2] is one such antacid. It reacts with hydrochloric acid in the stomach according to the following reaction:
Mg(OH)2 + 2HCl → MgCl2 + 2H2O
How many grams of HCl can a 200 mg dose of Mg(OH)2 neutralize?
-
Acid rain is caused by the reaction of nonmetal oxides with water in the atmosphere. One such reaction involves nitrogen dioxide (NO2) and produces nitric acid (HNO3):
3NO2 + H2O → 2HNO3 + NO
If 1.82 × 1013 g of NO2 enter the atmosphere every year due to human activities, potentially how many grams of HNO3 can be produced annually?
-
A simplified version of the processing of iron ore into iron metal is as follows:
2Fe2O3 + 3C → 4Fe + 3CO2
How many grams of C are needed to produce 1.00 × 109 g of Fe?
-
The SS Hindenburg contained about 5.33 × 105 g of H2 gas when it burned at Lakehurst, New Jersey, in 1937. The chemical reaction is as follows:
2H2 + O2 → 2H2O
How many grams of H2O were produced?
Answers
-
127 g
-
1.236 mol
-
23.9 g
-
0.251 g
-
1.61 × 108 g
(Back to the Top)
6.7 Chapter Summary
To ensure that you understand the material in this chapter, you should review the meanings of the following bold terms in the following summary and ask yourself how they relate to the topics in the chapter.
Chemical reactions relate quantities of reactants and products. Chemists use the mole unit to represent 6.022 × 1023 things, whether the things are atoms of elements or molecules of compounds. This number, called Avogadro’s number, is important because this number of atoms or molecules has the same mass in grams as one atom or molecule has in atomic mass units. Molar masses of substances can be determined by summing the appropriate masses from the periodic table; the final molar mass will have units of grams.
Because one mole of a substance will have a certain mass, we can use that relationship to construct conversion factors that will convert a mole amount into a mass amount, or vice versa. Such mole-mass conversions typically take one algebraic step.
Chemical reactions list reactants and products in molar amounts, not just molecular amounts. We can use the coefficients of a balanced chemical equation to relate moles of one substance in the reaction to moles of other substances (stoichiometry). In a mole-mass calculation, we relate the number of moles of one substance to the mass of another substance. In a mass-mass calculation, we relate the mass of one substance to the mass of another substance.
Additional Exercises
-
If the average male has a body mass of 70 kg, of which 60% is water, how many moles of water are in an average male?
-
If the average female is 60.0 kg and contains 0.00174% iron, how many moles of iron are in an average female?
-
How many moles of each element are present in 2.67 mol of each compound?
- HCl
- H2SO4
- Al(NO3)3
- Ga2(SO4)3
-
How many moles of each element are present in 0.00445 mol of each compound?
- HCl
- H2SO4
- Al2(CO3)3
- Ga2(SO4)3
-
What is the mass of one hydrogen atom in grams? What is the mass of one oxygen atom in grams? Do these masses have a 1:16 ratio, as expected?
-
What is the mass of one sodium atom in grams?
-
If 6.63 × 10−6 mol of a compound has a mass of 2.151 mg, what is the molar mass of the compound?
-
Hemoglobin (molar mass is approximately 64,000 g/mol) is the major component of red blood cells that transports oxygen and carbon dioxide in the body. How many moles are in 0.034 g of hemoglobin?
Answers
-
2,330 mol
-
- 2.67 mol of H and 2.67 mol of Cl
- 5.34 mol of H, 2.67 mol of S, and 10.68 mol of O
- 2.67 mol of Al, 8.01 mol of N, and 24.03 mol of O
- 5.34 mol of Ga, 8.01 mol of S, and 32.04 mol of O
-
H = 1.66 × 10−24 g and O = 2.66 × 10−23 g; yes, they are in a 1:16 ratio.
-
324 g/mol
(Back to the Top)
6.8 References:
Chapter 6 materials have been adapted and modified from the following creative commons resources unless otherwise noted:
1. Anonymous. (2012) Introduction to Chemistry: General, Organic, and Biological (V1.0). Published under Creative Commons by-nc-sa 3.0. Available at: http://2012books.lardbucket.org/books/introduction-to-chemistry-general-organic-and-biological/index.html
2. Poulsen, T. (2010) Introduction to Chemistry. Published under Creative Commons by-nc-sa 3.0. Available at: http://openedgroup.org/books/Chemistry.pdf
3. OpenStax (2015) Atoms, Isotopes, Ions, and Molecules: The Building Blocks. OpenStax CNX.Available at: http://cnx.org/contents/be8818d0-2dba-4bf3-859a-737c25fb2c99@12.